Backward elimination is an iterative model selection procedure that begins by considering the model that contains all
Question:
Backward elimination is an iterative model selection procedure that begins by considering the model that contains all of the potential independent variables and then attempts to remove independent variables one at a time from this model. On each step, an independent variable is removed from the model if it has the largest p-value of any independent variable remaining in the model and if its p-value is greater than αstay, an α value for allowing a variable to stay in the model. Backward elimination terminates when all the p-values for the independent variables remaining in the model are less than αstay. For example, Figure 15.38 shows the MINITAB output of a backward elimination for the residential sales data in Tables 15.17 and 15.19. Here, αstay has been set equal to .05. Interpret the steps in the output and write out the model that is found by the backward elimination procedure.
Data from Figure 15.38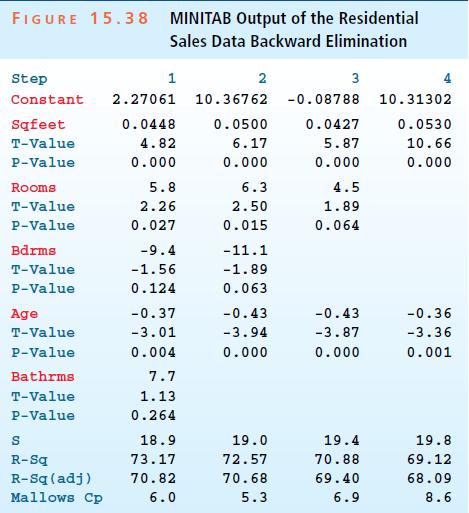
Data from Table 15.17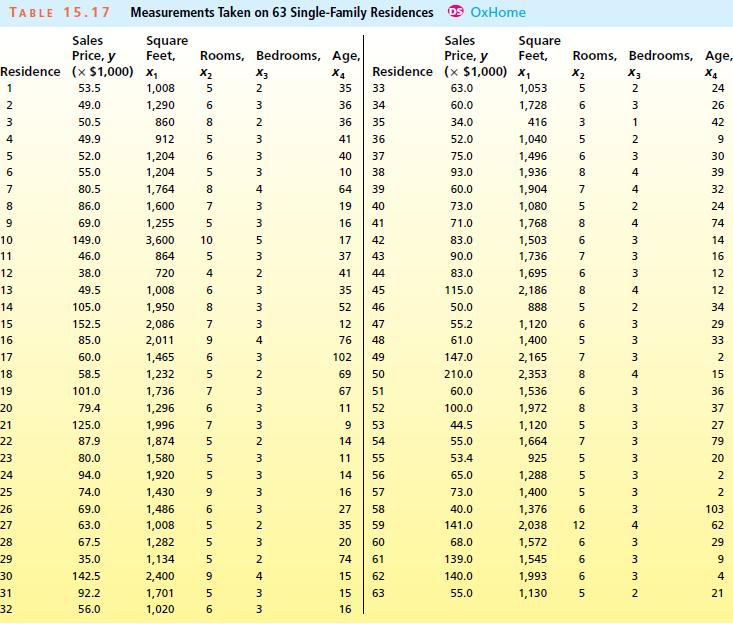
Data from Table 15.19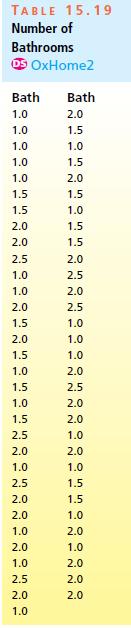
Step by Step Answer:

Business Statistics In Practice
ISBN: 9780077534844
7th Edition
Authors: Bruce Bowerman, Richard OConnell, Emilly Murphree





