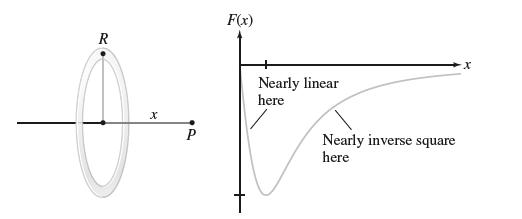
A narrow, negatively charged ring of radius R exerts a force on a positively charged particle P located at distance x above the center of the ring of magnitude

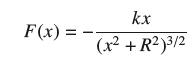
where k > 0 is a constant (Figure 10).

(a) Compute the third-degree Maclaurin polynomial for F.
(b) Show that F ≈ −(k/R³)x to second order. This shows that when x is small, F(x) behaves like a restoring force similar to the force exerted by a spring.
(c) Show that F(x) ≈ −k/x² when x is large by showing that

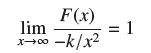
Thus, F(x) behaves like an inverse square law, and the charged ring looks like a point charge from far away.

Transcribed Image Text:

F(x)= kx (x² + R²)³/2