In the notation of Exercise 66, give an example where L exists but neither A nor B
Question:
In the notation of Exercise 66, give an example where L exists but neither A nor B exists.
Data From Exercise 66
Assume that the following limits exist:
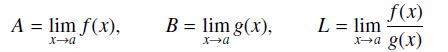
Prove that if L = 1, then A = B. You cannot use the Quotient Law if B = 0, so apply the Product Law to L and B instead.
Transcribed Image Text:
A = lim f(x), x→a B = lim g(x), x→a f(x) x→a g(x) L = lim
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 100% (3 reviews)
Suppose Then ...View the full answer

Answered By

Nazrin Ziad
I am a post graduate in Zoology with specialization in Entomology.I also have a Bachelor degree in Education.I posess more than 10 years of teaching as well as tutoring experience.I have done a project on histopathological analysis on alcohol treated liver of Albino Mice.
I can deal with every field under Biology from basic to advanced level.I can also guide you for your project works related to biological subjects other than tutoring.You can also seek my help for cracking competitive exams with biology as one of the subjects.
3.30+
2+ Reviews
10+ Question Solved
Related Book For 

Question Posted:
Students also viewed these Mathematics questions
-
Give an example where (f (x) + g(x)) exists but neither f(x) nor g(x) exists. lim
-
Fullerton Waste Management purchased land and a warehouse for $750,000. In addition to the purchase price, Fullerton made the following expenditures related to the acquisition: broker's commission,...
-
The FBIs Uniform Crime Reporting (UCR) program gathers crime data from more than 17,000 law enforcement agencies throughout the United States. The Crime data set contains crime counts for several...
-
In each of the homeowners forms, OA) the property coverage is the same OB) the liability coverage varies OC) both the property and liability coverage are the same OD) the property coverage varies
-
Many pay structures involve compensation combining both cash and stock. Why do firms want employees to be holders of the firm's common stock? What additional performance measurement and reward issues...
-
Bangers, Inc. is a start-up manufacturer of Australian-style frozen veggie pies located in San Antonio, Texas. The company is five years old and recently installed the manufacturing capacity to...
-
What are the three most common types of reports generated by an HRIS? What are their uses?
-
Suppose that the hourly output of chili at a barbecue (q, measured in pounds) is characterized by q = 20KL Where K is the number of large pots used each hour and L is the number of worker hours...
-
Review the following scenario. Use the information provided to answer questions about the taxpayer's 2022 return. Xavier Brown (32) is filing as a single taxpayer. During the year, he was unhappy...
-
True or false? (a) If lim f(x) exists, then lim f(x) = f(3). x-3 X-3 f(x) (b) If lim: = 1, then f(0) = 0. X 1 1 = x7 f(x) 8 (c) If lim f(x) = 8, then lim X-7' (d) If lim f(x) = 4 and lim f(x) = 8,...
-
Assume that the following limits exist: Prove that if L = 1, then A = B. You cannot use the Quotient Law if B = 0, so apply the Product Law to L and B instead. A = lim f(x), xa B = lim g(x), xa f(x)...
-
Last-minute revenue adjustments, unsupported balance sheet amounts, and improperly recorded revenues are examples of: a. Analytical symptoms. b. Documentary symptoms. c. Control symptoms. d....
-
X 10 Let A = -9 y 7 4 Z 210 If the kernel of A contains the vector what are x, y, and z? -2
-
8-22. E.O.Q., Carrying cost = Storing cost + Interest. Following data are available with respect to a certain material. Annual requirement.......... Cost to place an order.. Annual interest rate. _...
-
A new company started production. Job 1 was completed, and Job 2 remains in production. Here is the information from the job cost sheets from their first and only jobs so far: Job 1 Hours Total Cost...
-
1 Evaluate the given limits using the 10. f(x) = (x-3)(x-5) (a) lim f(x) X-3 (b) lim f(x) x-3+ (c) lim f(x) X-3 graph of the function (d) lim f(x) x+5= (e) lim f(x) x+5+ (f) lim f(x) X-5 50 y -50 2 6...
-
In Year 1 , Stallman Co . had a break - even point of 8 0 , 0 0 0 units, a selling price of $ 1 9 per unit, and fixed costs of $ 2 0 0 , 0 0 0 . What is Stallman Co . s variable cost per unit? (...
-
The law firm of Furlan and Benson accumulates costs associated with individual cases, using a job order cost system. The following transactions occurred during July: July 3. Charged 175 hours of...
-
Assume you are the accountant for Catalina Industries. John Catalina, the owner of the company, is in a hurry to receive the financial statements for the year ended December 31, 20X1, and asks you...
-
(a) If the symbol [ ] denotes the greatest integer function defined in Example 10, evaluate (b) If n is an integer, evaluate (c) For what values of does limxa [x] exist? lim, xl lim X lim x
-
(a) If the symbol [ ] denotes the greatest integer function defined in Example 10, evaluate (b) If n is an integer, evaluate (c) For what values of does limxa [x] exist? lim, xl lim X lim x
-
If f(x) = [x] + [-x], show that limx2 f(x) exists but is not equal to f(2).
-
If Novak Corp. has net sales of $ 523000 and cost of goods sold of $ 364000, Novak gross proftrate is O 100%. O 70%. 44%. 30%. Save for Later Attempts: 0
-
Required information The Hard Rock Mining Company is developing cost formulas for management planning and decision-making purposes. The company's cost analyst has concluded that utilities cost is a...
-
Leroy and Jenny (Married Filing Jointly) have two children ages 10 and 8 at home. In 2018 they paid $10,000 in daycare to their oldest daughter (21 years of age...and she is no longer claimed as a...

Study smarter with the SolutionInn App


