Let \(I\) be the flux of \(\mathbf{F}=\left\langle e^{y}, 2 x e^{x^{2}}, z^{2}ightangle\) through the upper hemisphere \(\mathcal{S}\) of the unit sphere.
(a) Let \(\mathbf{G}=\left\langle e^{y}, 2 x e^{x^{2}}, 0ightangle\). Find a vector field \(\mathbf{A}\) such that \(\operatorname{curl}(\mathbf{A})=\mathbf{G}\).
(b) Use Stokes' Theorem to show that the flux of \(\mathbf{G}\) through \(\mathcal{S}\) is zero. Calculate the circulation of \(\mathbf{A}\) around \(\partial \mathcal{S}\).

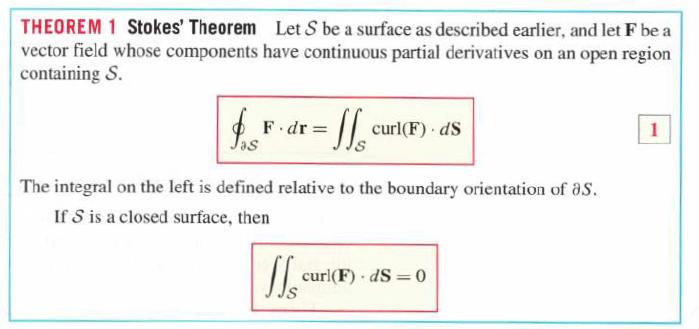
(c) Calculate I. Use (b) to show that \(I\) is equal to the flux of \(\left\langle 0,0, z^{2}ightangle\) through \(\mathcal{S}\).

Transcribed Image Text:

THEOREM 1 Stokes' Theorem Let S be a surface as described earlier, and let F be a vector field whose components have continuous partial derivatives on an open region containing S. ko = JS F.dr: curl(F). dS The integral on the left is defined relative to the boundary orientation of aS. If S is a closed surface, then Ja curl(F) dS=0 S . 1