Show that \(\Phi(\theta, \phi)=(a \cos \theta \sin \phi, b \sin \theta \sin \phi, c \cos \phi)\) is a parametrization of the ellipsoid
\[
\left(\frac{x}{a}ight)^{2}+\left(\frac{y}{b}ight)^{2}+\left(\frac{z}{c}ight)^{2}=1
\]
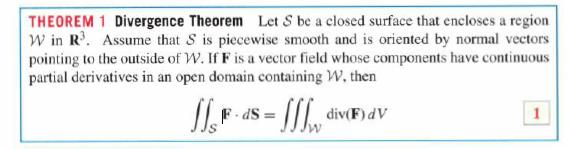
Then calculate the volume of the ellipsoid as the surface integral of \(\mathbf{F}=\frac{1}{3}\langle x, y, zangle\) (this surface integral is equal to the volume by the Divergence Theorem).

THEOREM 1 Divergence Theorem Let S be a closed surface that encloses a region W in R. Assume that S is piecewise smooth and is oriented by normal vectors pointing to the outside of W. If F is a vector field whose components have continuous partial derivatives in an open domain containing W, then 1. ds = [/ []] F-ds: div(F) dv 1