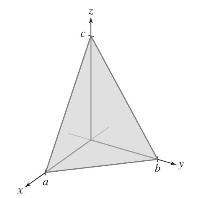
Show that the \(z\)-coordinate of the centroid of the tetrahedron bounded by the coordinate planes and the plane

\[\frac{x}{a}+\frac{y}{b}+\frac{z}{c}=1\]

in Figure 14 is \(\bar{z}=c / 4\). Conclude by symmetry that the centroid is \((a / 4, b / 4, c / 4)\).

Transcribed Image Text:

a C b