The torque about the origin O due to a force F acting on an object with position
Question:
The torque about the origin O due to a force F acting on an object with position vector r is the vector quantity τ = r × F. If several forces Fj act at positions rj, then the net torque (units: N-m or lb-ft) is the sum
5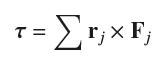
Continuing with Exercise 69, suppose that L1 = 3 m, L2 = 2 m, m1 = 15 kg, m2 = 20 kg, and m3 = 18 kg. If the angles θ1, θ2 are equal (say, to θ), what is the maximum allowable value of θ if we assume that the robotic arm can sustain a maximum torque of 1200 N-m?
Data From Exercise 69
Let τ be the net torque about O acting on the robotic arm of Figure 22, here taking into account the weight of the arms themselves. Assume that the arms have mass m1 and m2 (in kilograms) and that a weight of m3 kg is located at the endpoint P. In calculating the torque, we may assume that the entire mass of each arm segment lies at the midpoint of the arm (its center of mass). Show that the position vectors of the masses m1, m2, and m3 are
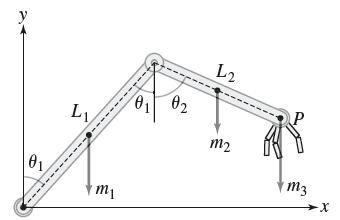
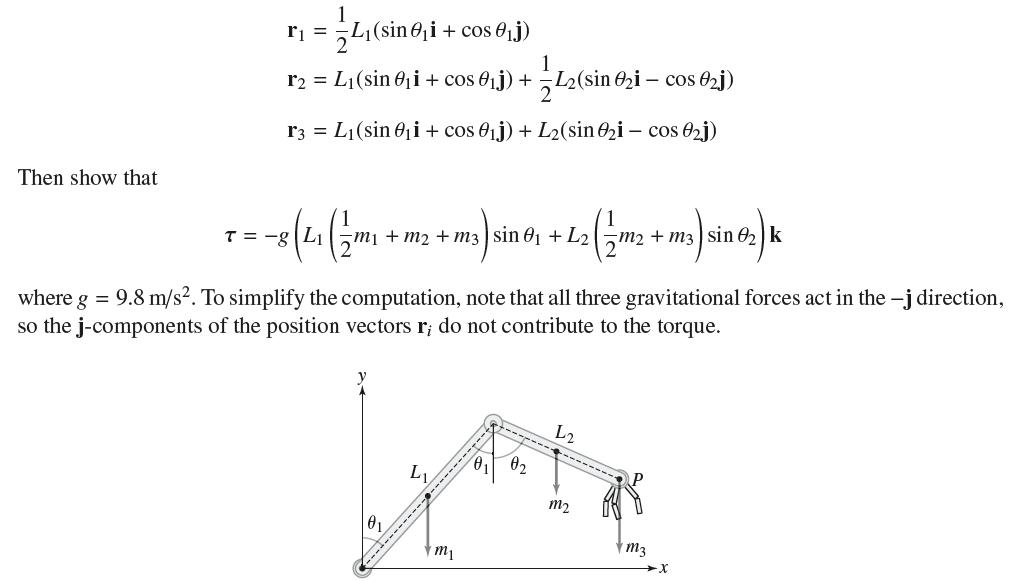
Step by Step Answer:






