A cylinder of density o , length l, and cross-section area A floats in a liquid

A cylinder of density ρ_o , length l, and cross-section area A floats in a liquid of density ρ_f with its axis perpendicular to the surface. Length h of the cylinder is submerged when the cylinder floats at rest.

a. Show that h = (ρ_o/ρ_f)l.

b. Suppose the cylinder is distance y above its equilibrium position. Find an expression for (F_net)_y, the y-component of the net force on the cylinder. Use what you know to cancel terms and write this expression as simply as possible.

c. You should recognize your result of part b as a version of Hooke’s law. What is the “spring constant” k?

d. If you push a floating object down and release it, it bobs up and down. So it is like a spring in the sense that it oscillates if displaced from equilibrium. Use your “spring constant” and what you know about simple harmonic motion to show that the cylinder’s oscillation period is

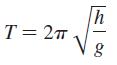
e. What is the oscillation period for a 100-m-tall iceberg (ρ_ice = 917 kg/m³) in seawater?

Transcribed Image Text:

T= 2n