A projectile is launched from the ground with an initial speed V at an angle from

A projectile is launched from the ground with an initial speed V at an angle θ from the horizontal. Assume that the x-axis is the horizontal ground and y is the height above the ground. Neglecting air resistance and letting g be the acceleration due to gravity, it can be shown that the trajectory of the projectile is given by

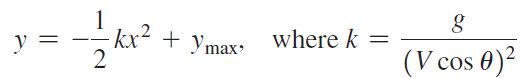 and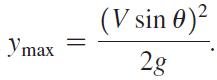

a. Note that the high point of the trajectory occurs at (0, y_max). If the projectile is on the ground at (-a, 0) and (a, 0), what is a?

b. Show that the length of the trajectory (arc length) is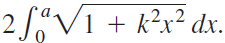

c. Evaluate the arc length integral and express your result in terms of V, g, and θ.

d. For a fixed value of V and g, show that the launch angle θ that maximizes the length of the trajectory satisfies (sin θ) ln (sec θ + tan θ) = 1.

e. Use a graphing utility to approximate the optimal launch angle.

Transcribed Image Text:

1 kx- + ymax, where k y = %3| (V cos 0)² (V sin 0)? y max 2g