Let I_n = ∫xⁿe^-x2 dx, where n is a nonnegative integer. 

a. I₀ = ∫ e^-x2 dx cannot be expressed in terms of elementary functions. Evaluate I₁.    

b. Use integration by parts to evaluate I₃.

c. Use integration by parts and the result of part (b) to evaluate I₅.

d. Show that, in general, if n is odd, then 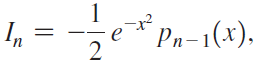where p_{n - 1} is a polynomial of degree n - 1.

e. Argue that if n is even, then In cannot be expressed in terms of elementary functions.

4 = * Pa-1(*), u.