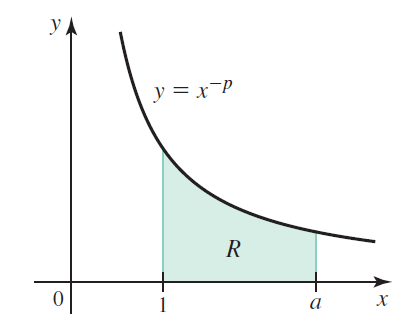
Let R be the region bounded by y = 1/x^p and the x-axis on the interval [1, a], where p > 0 and a > 1 (see figure). Let V_x and V_y be the volumes of the solids generated when R is revolved about the x- and y-axes, respectively.

a. With a = 2 and p = 1, which is greater, V_x or V_y?

b. With a = 4 and p = 3, which is greater, V_x or V_y?

c. Find a general expression for Vx in terms of a and p. Note that p = 1/2 is a special case. What is V_x when p = 1/2?

d. Find a general expression for V_y in terms of a and p. Note that p = 2 is a special case. What is V_y when p = 2?

e. Explain how parts (c) and (d) demonstrate that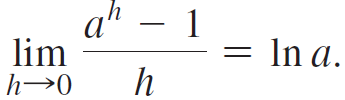

f. Find any values of a and p for which V_x > V_y.

Transcribed Image Text:

a' lim - 1 In a. УА y, у—х Р х