One of several Leibniz Rules in calculus deals with higher-order derivatives of products. Let (fg)⁽ⁿ⁾ denote the nth derivative of the product fg, for n ≥ 1.

a. Prove that (fg)⁽²⁾ = f"g + 2 f'g' + fg".

b. Prove that, in general,

where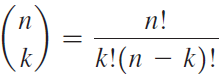are the binomial coefficients.

c. Compare the result of (b) to the expansion of (a + b)ⁿ.

Transcribed Image Text:

п п f(k)g(n=k). |(f8)(") Σ k=0 п п! k) k.(п — К)!