Suppose f is differentiable on an interval containing a and b, and let P(a, f(a)) and Q(b, f(b)) be distinct points on the graph of f. Let c be the x-coordinate of the point at which the lines tangent to the curve at P and Q intersect, assuming that the tangent lines are not parallel (see figure).

a. If f(x) = x², show that c = (a + b)/2, the arithmetic mean of a and b, for real numbers a and b.

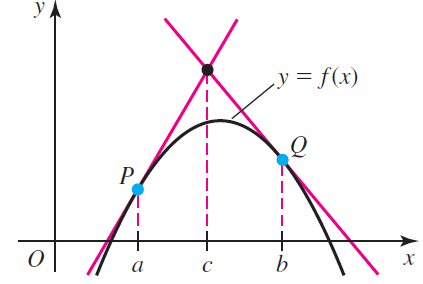
b. If f(x) = √x, show that c = √ab, the geometric mean of a and b, for a > 0 and b > 0.

c. If f(x) = 1/x, show that c = 2ab/(a + b), the harmonic mean of a and b, for a > 0 and b > 0.

d. Find an expression for c in terms of a and b for any (differentiable) function f whenever c exists.

y = f(x)