Suppose the functions f and g are continuous on a, b and differentiable on (a, b), where g(a) g(b) Then there is a point c in (a, b) at which This result is known as the Generalized (or Cauchys) Mean Value Theorem a If g(x) x, then show that the Generalized Mean Value Theorem reduces to the Mean Va...

Suppose the functions f and g are continuous on [a, b] and differentiable on (a, b), where

Question:

Suppose the functions f and g are continuous on [a, b] and differentiable on (a, b), where g(a) ≠ g(b). Then there is a point c in (a, b) at which

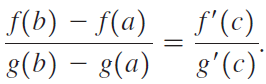
f(b) – f(a) _ f'(c) g(b) – 8(a) g'(c)'

This result is known as the Generalized (or Cauchy’s) Mean Value Theorem.

a. If g(x) = x, then show that the Generalized Mean Value Theorem reduces to the Mean Value Theorem.

b. Suppose f(x) = x² - 1, g(x) = 4x + 2, and [a, b] = [0, 1]. Find a value of c satisfying the Generalized Mean Value Theorem.

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Calculus Early Transcendentals

ISBN: 978-0321947345

2nd edition

Authors: William L. Briggs, Lyle Cochran, Bernard Gillett

See More Books

Question Posted: Mar 05, 2019 07:53 AM

See More Questions

If f and g are continuous on [a, b], then 9, SLS)g(x)] dx g(x) dx %3| f(x) dx
If f and g are continuous on [a, b] and if ba f = ba g, prove that there exists c [a, b] such that f(c) = g(c).
Functions f and g are defined on R by f (x) := x + 1 and g (x) := 2 if x 1 and g(1) := 0. lim g (f(x) g (lim f(x))
A major electronics manufacturer expects to generate additional revenue from its recently won government contract. The company forecasts that the revenue will be $190 million in the first year, but...
Ashley Limited, which follows ASPE, had the following information available at the end of 2014: Note 1: Investment income for the trading investments (FV-NI) includes dividend income of $2,400 and a...
How would a manufacturers strategy for choosing a retailer change depending on its overall market power and the consistency of the new product with existing offerings?
5. On January 2, 2016, Pam Corporation bought 15 percent of Sun Corporations capital stock for $30,000. Pam accounts for this investment using the cost method. Suns net income for the years ended...
Complete the table below for contribution margin per unit, total contribution margin, and contribution margin ratio: Number of units Sales price per unit Variable costs per unit Calculate:...
29) Value stocks are more likely to have a PE ratio _____. Multiple Choice A) less than 1 B) equal to 1 C) greater than 1 D) None of the above.
Mr B aged 52 years, has earned rupees 75,00,000 out of his business. His ex-wife gifted him a car worth rupees 8 lakh. He spent a total of rupees 20 lakh during a family trip. He won a lottery of 16...
Evaluate the following limits. Use lHpitals Rule when needed. lim 20 cot 30
A crank of radius r rotates with an angular frequency v. It is connected to a piston by a connecting rod of length L (see figure). The acceleration of the piston varies with the position of the crank...
Describe other factors that Lee must take into account as she considers capacity expansion options.
Sketch the requested conic sections in Problems 14-23 using the definition. A parabola with the distance between the directrix and focus 1 unit
Why is the Rosenblum case a particularly important case in auditor liability?
Draw a population curve for a city whose growth rate is $1.3 \%$ and whose present population is 53,000 . The equation is \[P=P_{0} e^{r t}\] Let $t=0,10, \cdots, 50$ to help you find points for...
Draw a bar graph for each data set in Problems 32-35. Data set B Data set A: The annual wages of employees at a small accounting firm are given in thousands of dollars. 35 25 25 16 14 1 2 25 18 2 2...
For the four unrelated situations, A-D, below, calculate the unknown amounts indicated by the letters appearing in each column: B D Beginning Assets... Liabilities.. $40,000 $12,000 $28,000 $ (d)...
On 1 July 2015, Shark Ltd purchased three machines, each used in a different production process in the factory. On 30 June 2016, there was an indication that the machines could be impaired due to a...
You are thinking of investing in one of two companies. In one annual report, the auditors opinion states that the financial statements were prepared in accordance with generally accepted accounting...
Use (a) The Trapezoidal Rule (b) The Midpoint Rule (c) Simpsons Rule with n = 10 to approximate the given integral. Round your answers to six decimal places. SVi cos. cos x dx
Evaluate the integral. sin 2x 1+ cos*x
The Cauchy principal value of the integral defined by Show that diverges but the Cauchy principal value of this integral is 0. f(x) dx -00
You borrowed $15,000 for buying a new car from a bank at an interest rate of 12% compounded monthly. This loan will be repaid in 48 equal monthly installments over four years. Immediately after the...
Discuss how debt restructuring, settlement, or modification works. Discuss the journal entries for debtor and creditor
Could CNL be a viable business? If so, under what conditions and what level of production (and, since production is directly related to production workers, employees)? All information provided for...

Suppose the functions f and g are continuous on [a, b] and differentiable on (a, b), where

Question:

f(b) – f(a) _ f'(c) g(b) – 8(a) g'(c)'

Step by Step Answer:

a If gx x the...View the full answer

Calculus Early Transcendentals

Students also viewed these Mathematics questions