You must get from a point P on the straight shore of a lake to a stranded swimmer who is 50 m from a point Q on the shore that is 50 m from you (see figure). If you can swim at a speed of 2 m/s and run at a speed of 4 m/s, at what point along the shore, x meters from Q, should you stop running and start swimming if you want to reach the swimmer in the minimum time?

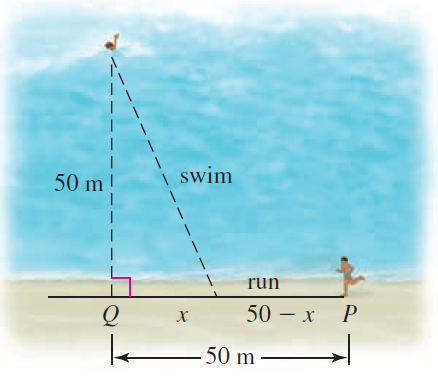
a. Find the function T that gives the travel time as a function of x, where 0 ≤ x ≤ 50.

b. Find the critical point of T on (0, 50).

c. Evaluate T at the critical point and the endpoints (x = 0 and x = 50) to verify that the critical point corresponds to an absolute minimum. What is the minimum travel time?

d. Graph the function T to check your work.

Transcribed Image Text:

i swim 50 m! run 50- х Р х 50 m