Each of Exercises 81 through 85 involves either the chain rule for partial derivatives or the incremental
Question:
Each of Exercises 81 through 85 involves either the chain rule for partial derivatives or the incremental approximation formula for functions of two variables.
Use the formula obtained in Exercise 84 to find the slope of the level curve x2 + xy + y3 = 1 at the point (−1, 1). What is the equation of the tangent line to the level curve at this point?
Data from Exercises 84
Suppose y = h(x) is a differentiable function of x and that F(x, y) = C for some constant C. Use the chain rule (with x taking the role of t) to show that
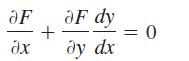
Conclude that the slope at each point (x, y) on the level curve F(x, y) = C is given by
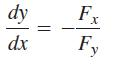
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Calculus For Business, Economics And The Social And Life Sciences
ISBN: 9780073532387
11th Brief Edition
Authors: Laurence Hoffmann, Gerald Bradley, David Sobecki, Michael Price
Question Posted:





