Exercises refer to the Laffer curve, originated by the economist Arthur Laffer. An idealized version of this curve is shown here. According to this curve, decreasing a tax rate, say from x₂ percent to x₁ percent on the graph, can actually lead to an increase in government revenue. The theory is that people will work harder and earn more money if they are taxed at a lower rate, so the government ends up with more revenue than it would at a higher tax rate. All economists agree on the endpoints—0 revenue at tax rates of both 0% and 100%—but there is much disagreement on the location of the tax rate x₁ that produces the maximum revenue.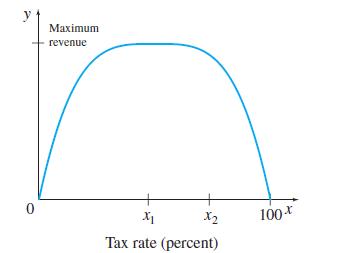

A function that might describe the entire Laffer curve is y = x(100 - x)(x² + 500), where y is government revenue in hundreds of thousands of dollars from a tax rate of x percent, with the function valid for 0 ≤ x ≤ 100. Find the revenue from the following tax rates.
(a) 10% 

(b) 40% 

(c) 50% 

(d) 80%
(e) Graph the function.

Transcribed Image Text:

0 Maximum revenue x1 X2 Tax rate (percent) 100x