To show that two perpendicular lines, neither of which is vertical, have slopes with a product of
Question:
To show that two perpendicular lines, neither of which is vertical, have slopes with a product of -1, go through the following steps. Let line L1 have equation y = m1 x + b1 , and let L2 have equation y = m2x + b2, with m1 > 0 and m2 < 0. Assume that L1 and L2 are perpendicular, and use right triangle MPN shown in the figure. Prove each of the following statements.
(a) MQ has length m1 .
(b) QN has length -m2 .
(c) Triangles MPQ and PNQ are similar.
(d) m1/1 = 1/(-m2) and m1 m2 = -1
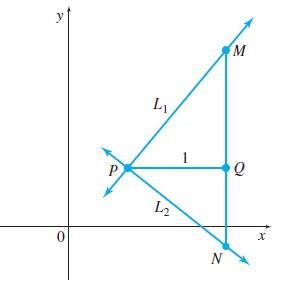
Transcribed Image Text:
0 L₁ L2 1 N M Q x
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 33% (6 reviews)
a See the figure in the textbook Segment MN is drawn perpendicular to segme...View the full answer

Answered By

Ajeet Singh
Professional, Experienced, and Expert tutor who will provide speedy and to-the-point solutions.
I have been teaching students for 5 years now in different subjects and it's truly been one of the most rewarding experiences of my life.
I have also done one-to-one tutoring with 100+ students and help them achieve great subject knowledge.
I have expertise in computer subjects like C++, C, Java, and Python programming and other computer Science related fields.
Many of my student's parents message me that your lessons improved their children's grades and this is the best only thing you want as a teacher. I have done plenty of studying and lots of learning on many exciting and challenging topics. All these experiences have influenced my decision to take on the teaching role in various capacities. As a tutor, I am looking forward to getting to understand your needs and helping you achieve your academic goals. I'm highly flexible and contactable. I am available to work on short notice since I only prefer to work with very small and select groups of students.
4.90+
7+ Reviews
15+ Question Solved
Related Book For 

Question Posted:
Students also viewed these Mathematics questions
-
In general, it is difficult to show that two matrices are similar. However, if two similar matrices are diagonalizable, the task becomes easier. In Exercises 1-2 show that A and are similar by...
-
In general, it is difficult to show that two matrices are similar. However, if two similar matrices are diagonalizable, the task becomes easier. Show that A and B are similar by showing that they are...
-
In general, it is difficult to show that two matrices are similar. However, if two similar matrices are diagonalizable, the task becomes easier. Show that A and B are similar by showing that they are...
-
Carol Simon, the manager of Handy Plumbing has provided you with the following aging schedule for Handys accounts receivable: Carol indicates that the $125,200 of accounts receivable identified in...
-
Refer to Case Study 21.1, illustrating the role of calcium in reducing the symptoms of PMS. Using the caution given at the end of the case study, explain why we cannot use the method presented in...
-
In this chapter, group rules and norms are stressed as being very important to effective teams. Do you agree with this? Explain your answer. Briefly comment on your own desire and ability to adapt to...
-
3. How are previously held investments revalued in a piecemeal acquisition?
-
Cash Flow Intuition A project has an initial cost of 1, has required return of R, and pays C annually for N years. a. Find C in terms of l and N such that the project has a payback period just equal...
-
Required information [The following information applies to the questions displayed below] York's outstanding stock consists of 70,000 shares of noncumulative 7.5% preferred stock with a $5 par value...
-
Us Republic Corporation Balance Sheet, December 31, 20x3 Us Republic Corporation Statement Of Income And Retained Earnings, Year Ended December 31, 20x3 a. Fill in the 20X3 column in the table that...
-
Consider the equation (a) Show that this equation represents a line by writing it in the form y = mx + b. (b) Find the x- and y-intercepts of this line. (c) Explain in your own words why the equation...
-
The following data give the expected points for a football team with first down and 10 yards to go from various points on the field. (a) Calculate the correlation coefficient. Does there appear to be...
-
What is the typical noncash item, by name, that is automatically added back in the operating activities section of the SCF? LO1
-
A parent acquires all of the stock of a subsidiary for $40 million in cash. The subsidiarys books report the following account balances at the date of acquisition (in trial balance format)....
-
1. Given: The sign for the Inn of the Prancing Pony in Bree-yes, it comes in pints-is fixed on the end of a beam of length 5L. If the sigh deflects too much then Gandalf will hit his head when he...
-
Q21) Add positive and negative charges as shown in the diagram below. Use the arrows of the simulation to guide you in drawing continuous electric field lines around and in between the three charges....
-
When 10.1 g CaO is dropped into a styrofoam coffee cup containing 157 g H2O at 18.0C, the temperature rises to 35.8C. Calculate the enthalpy change of the following reaction in kJ/mol CaO. Assume...
-
4-12. Sometimes heterogeneous chemical reactions take place at the walls of tubes in which reactive mixtures are flowing. If species A is being consumed at a tube wall because of a chemical reaction,...
-
The Golden Gate Bridge is a famous suspension bridge in San Francisco. The main segment is approximately 1400 m long and is composed of steel. Estimate how much the length of the bridge increases as...
-
As long as we can't lose any money, we have a risk-free investment." Discuss this comment. Q2: Both investing and gambling can be defined as "undertaking risk in order to earn a profit." Explain how...
-
Find each sum or difference. Write answers in standard form. (3 + 2i) + (9 - 3i)
-
Solve each formula for the specified variable. Assume that the denominator is not 0 if variables appear in the denominator. S = 2lw + 2wh + 2hl, for h (surface area of a rectangular box)
-
Solve each formula for the specified variable. Assume that the denominator is not 0 if variables appear in the denominator. for x (standardized value)
-
Saly paid $52,000 a year paid on a weekly basis. last pay she had $250 withheld in Income Tax, $48.97 for CPP and $15.80 for EI. an additional $and 25.00 in tax are deducted each pay. She allowed to...
-
Required information [The following information applies to the questions displayed below.] Dain's Diamond Bit Drilling purchased the following assets this year. Asset Drill bits (5-year) Drill bits...
-
Which of the following partnership items are not included in the self-employment income calculation? Ordinary income. Section 179 expense. Guaranteed payments. Gain on the sale of partnership...

Study smarter with the SolutionInn App


