Refer to Problem 3.27. The sample size may need to be quite large for the sampling distribution
Question:
Refer to Problem 3.27. The sample size may need to be quite large for the sampling distribution of γ̂ to be approximately normal, especially if |γ| is large. The Fisher-type transform ξ̂ = 1/2 log[(1 + γ̂)/(1 − γ̂)] converges more quickly to normality.
a. Show that the asymptotic variance of ξ̂ equals the asymptotic variance of γ̂ multiplied by (1 − γ2)?2.
b. Explain how to construct a confidence interval for ξ and use it to obtain one for γ.
c. Show that ξ̂ = 1/2 log(C/D). For 2 × 2 tables, show that this is half the log odds ratio.
Data from Problem 3.27:
For ordinal variables, consider gamma (2.14). Let
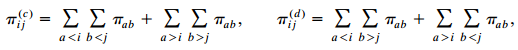
Where i and j are fixed in the summations. Show that IIc = ∑i ∑j πij π(c)ij and IId = ∑i ∑j πij π(d)ij. Use the delta method to show that the large-sample normality (3.9) applies for γ̂ , with (Goodman and Kruskal 1963)
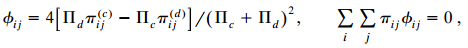
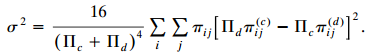
The word "distribution" has several meanings in the financial world, most of them pertaining to the payment of assets from a fund, account, or individual security to an investor or beneficiary. Retirement account distributions are among the most...
Step by Step Answer:






