Refer to Problem 3 42 and exact tests using X 2 with H Icirc plusmn Iuml 1 Iuml 2 Explain why the unconditional P value, evaluated at Iuml 0 5, is related to Fisher conditional P values for various tables by Thus, the unconditional P value of 1 32 is a weighted average of the Fisher P value for the...

The observed table has X 2 6 The probabi ...

Refer to Problem 3.42 and exact tests using X 2 with HÎ±: Ï 1 Ï 2 .

Question:

Refer to Problem 3.42 and exact tests using X²with HÎ±: Ï€₁‰ Ï€₂. Explain why the unconditional P-value, evaluated at Ï€ = 0.5, is related to Fisher conditional P-values for various tables by

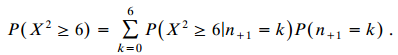
$P(X² > 6) = E P(X² > 6\n+1 = k)P(n+ = k) . k=0$

Thus, the unconditional P-value of 1/32 is a weighted average of the Fisher P-value for the observed column margins and P-values of 0 corresponding to the impossibility of getting results as extreme as observed if other margins had occurred i.e.

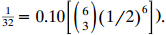
- 0.10 (8)(1/2)*), %3D 32

The Fisher quote in Section 3.5.6 gave his view about this.

Data from Prob. 3.42:

A contingency table for two independent binomial variable has counts (3, 0 / 0, 3) by row. For H₀: Ï€₁ = Ï€₂ and H_Î± : Ï€₁ > Ï€₂, show that the P-value equals 1/64 for the exact unconditional test and 1/20 for Fisher€™s exact test.