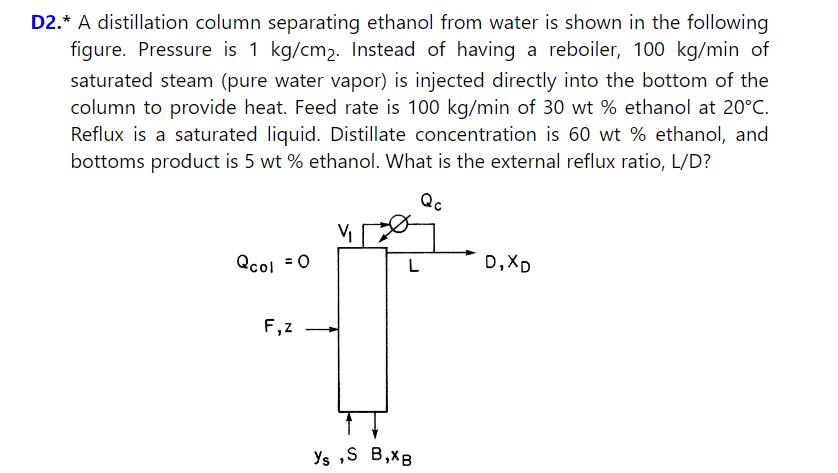
\(100 \mathrm{kmol} / \mathrm{h}\) of a \(60 \mathrm{~mol} \%\) methanol and \(40 \mathrm{~mol} \%\) water feed at \(40^{\circ} \mathrm{C}\) is distilled in a column that does not have a reboiler but uses open steam for heating (see figure in Problem 3.D2). The column is at \(1.0 \mathrm{~atm}\). The steam is pure water vapor \(\left(\mathrm{y}_{M}=0\right)\) and is a saturated vapor. The distillate product is \(99.0 \mathrm{~mol} \%\) methanol and is a saturated liquid. The bottoms is \(2.0 \mathrm{~mol} \%\) methanol and, because it leaves an equilibrium stage, must be a saturated liquid. The column is adiabatic and has a total condenser. External reflux ratio is \(\mathrm{L} / \mathrm{D}=2.3\). Find \(\mathrm{D}, \mathrm{B}, \mathrm{Q}_{\mathrm{c}}\), and \(\mathrm{S}\). Be careful with units and in selecting basis for energy balance.

Data: \(\lambda_{\text {methanol }}=\Delta \mathrm{H}_{\text {vap }}=8.43 \mathrm{kcal} / \mathrm{mol}=35.27 \mathrm{~kJ} / \mathrm{mol}\) (at boiling point)

\(\lambda_{\text {water }}=\Delta \mathrm{H}_{\text {vap }}=9.72 \mathrm{kcal} / \mathrm{mol}=40.656 \mathrm{~kJ} / \mathrm{mol}\) (at boiling point)

\(\mathrm{C}_{\mathrm{p}, \mathrm{w}, \text { liquid }}=1.0 \mathrm{cal} /\left(\mathrm{g}{ }^{\circ} \mathrm{C}\right)=75.4 \mathrm{~J} / \mathrm{mol}^{\circ} \mathrm{C}\)

\(\mathrm{C}_{\mathrm{PL}, \mathrm{Meoh}}=75.86+0.1683 T \mathrm{~J} /\left(\mathrm{mol}{ }^{\circ} \mathrm{C}\right)\)

\(\mathrm{C}_{\mathrm{p}, \mathrm{w}, \text { vapor }}=33.46+0.006880 \mathrm{~T}+0.7604 \times 10^{-5} \mathrm{~T}^{2}-3.593 \times 10^{-9} \mathrm{~T}^{3}\)

\(\mathrm{C}_{\mathrm{p}, \text { meoh,vapor }}=42.93+0.08301 \mathrm{~T}-1.87 \times 10^{-5} \mathrm{~T}^{2}-8.03 \times 10^{-9} \mathrm{~T}^{3}\)

\(\mathrm{T}\) is in \({ }^{\circ} \mathrm{C}, \mathrm{C}_{\mathrm{p}, \text { vapor }}\) values in \(\mathrm{J} / \mathrm{mol}{ }^{\circ} \mathrm{C}\)

Transcribed Image Text:

D2.* A distillation column separating ethanol from water is shown in the following figure. Pressure is 1 kg/cm2. Instead of having a reboiler, 100 kg/min of saturated steam (pure water vapor) is injected directly into the bottom of the column to provide heat. Feed rate is 100 kg/min of 30 wt % ethanol at 20C. Reflux is a saturated liquid. Distillate concentration is 60 wt % ethanol, and bottoms product is 5 wt % ethanol. What is the external reflux ratio, L/D? Qc Qcol = 0 L D,XD F,z Ys,S B,XB