A mixture of \(\beta\)-methylnaphthalene \(+\beta\)-chloronaphthalene \((\beta \mathrm{cn})\) at \(1.0 \mathrm{~atm}\) (Figure 17 \(8 \mathrm{~A})\) has liquid and solid in equilibrium at \(50^{\circ} \mathrm{C}\).

Figure (17-8A)

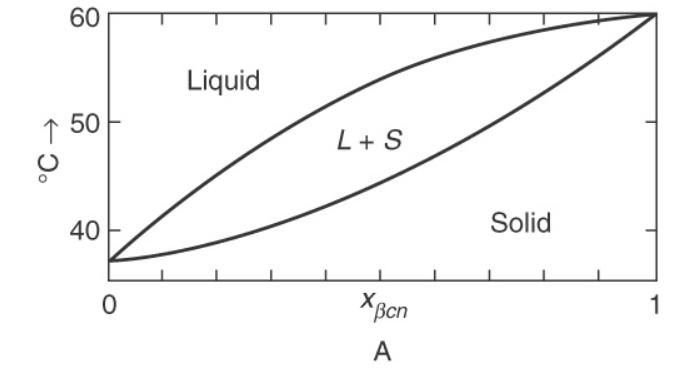
a. What are the mole fractions \(\left(\mathrm{x} \beta_{\mathrm{cn}}\right)\) of the liquid and solid phases in equilibrium?

b. What ranges of initial mole fraction can produce an equilibrium mixture at \(50^{\circ} \mathrm{C}\) ?

c. If the initial value of \(x \beta_{\mathrm{cn}}=0.5\) and the liquid and solid are in equilibrium at \(50^{\circ} \mathrm{C}\), what fraction of the original feed is liquid?

d. If the liquid in parts a and \(\mathrm{c}\) is removed and then cooled to \(45^{\circ} \mathrm{C}\), what are the mole fractions of the liquid and solid in equilibrium?

e. If the liquid in parts a and \(\mathrm{c}\) is removed and then cooled to \(35^{\circ} \mathrm{C}\), what is the average mole fraction of the solid?

Transcribed Image Text:

60 60 50 Liquid L+ S O 40 0 X Bcn A Solid 1