Complete the solutions for \(v_{1}\) in Example 14.4 for domain perturbation in the text. What are the boundary conditions for the \(v_{2}\) problem?

Example 14.4:

Consider flow in a rectangular channel. Let the walls be located at y = ±h, with y = 0 being the center of the walls. A pressure gradient G is applied in the x-direction and we have the classical plane Poiseuille flow problem with a parabolic velocity profile. This represents the base problem. Let us now consider the flow in a channel with a corrugated boundary with some sinusoidal corrugation in the z-direction. The geometry is shown in Fig. 14.5.

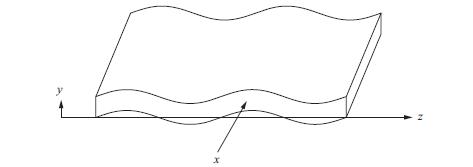
Figure 14.5 A figure showing the flow geometry considered in the domain perturbation problem. The gap width y is assumed to vary as a sinusoidal function of z. The flow direction is x.
The no-slip boundary condition is to be applied at

rather than at y = h.
The channel is corrugated in the z-direction and the flow is in the x-direction as indicated in Fig. 14.5. The symmetry condition holds at y = 0. In view of the symmetry, only the domain 0 ≤ y ≤ h need be considered.

Fig. 14.5: