Develop a spreadsheet program for a rectifying column. The feed is 32.0 \(\mathrm{mol} \% \mathrm{n}\)-butane, \(56.0 \mathrm{~mol} \% \mathrm{n}\)-pentane, and \(12.0 \mathrm{~mol} \% \mathrm{n}\)-hexane. The feed rate is \(100.0 \mathrm{kmol} / \mathrm{h}\) of a saturated vapor at \(7.0 \mathrm{bar}\). The column is at \(7.0 \mathrm{bar}\), has a total condenser with saturated liquid reflux, and CMO is valid. Distillate product is \(99.6 \mathrm{~mol} \% \mathrm{n}\)-butane, and \(\mathrm{D}=15.0 \mathrm{kmol} / \mathrm{h}\). The spreadsheet program in Figure 5-A1 and Table 5-A2 can be modified for rectifying columns. In a rectifying column, we can accurately estimate the mole fractions at the bottom of the column where the saturated vapor feed enters. First, change the input data to match this problem and change the preliminary calculations to match a rectifier. Next, make the changes necessary given that we must step off stages from the bottom up. The \(\mathrm{x}\) values are determined from the top operating line and \(y\) values from a bubble-point calculation. The working register holds \(\mathrm{x}\) values for calculation of the \(\mathrm{y}\) values from equilibrium for the next stage up the column. Fortunately, Eq. (2-28) is still useful to determine \(\mathrm{K}\) values. Because more changes are needed in the spreadsheet, this problem is more challenging than the other spreadsheet problems.

Equation (2-28)

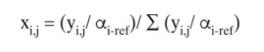
Figure 5-A1

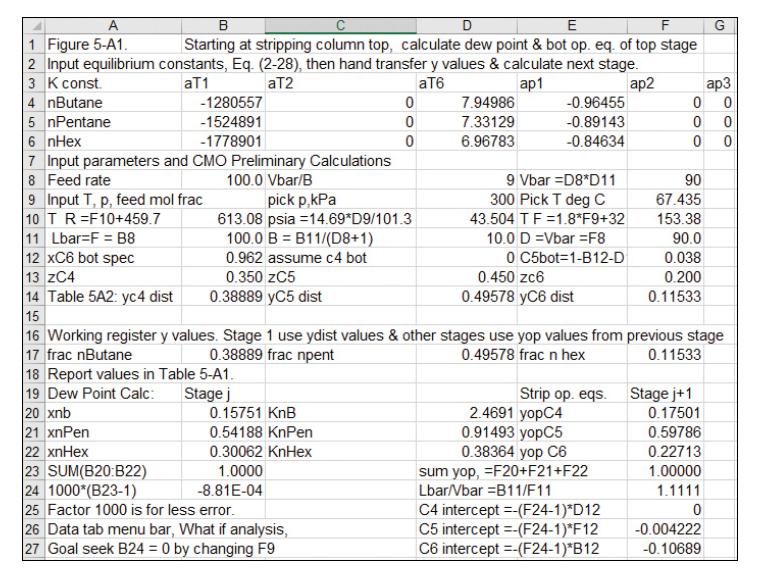
Table 5-A2

a. Modify the spreadsheet in Figure A-1 and Table A-2 for a rectifying column.

b. Find \(\mathrm{B}, \mathrm{L}_{0} / \mathrm{D}\), and distillate and bottoms mole fractions.

c. Find the number of stages and the temperature and mole fraction profiles.

Transcribed Image Text:

= Xij (yiji-ret)/(Yijai-ref)