Next, a particle with the same mass and velocity as the particle in Figure P24.59 enters the
Question:
Next, a particle with the same mass and velocity as the particle in Figure P24.59 enters the velocity selector. This particle has a charge of \(2 q\)-twice the charge of the particle in Figure P24.59. In this case, we can say that
A. The force of the electric field on the particle is greater than the force of the magnetic field.
B. The force of the magnetic field on the particle is greater than the force of the electric field.
C. The forces of the electric and magnetic fields on the particle are still equal.
In experiments where all the charged particles in a beam are required to have the same velocity (for example, when entering a mass spectrometer), scientists use a velocity selector. A velocity selector has a region of uniform electric and magnetic fields that are perpendicular to each other and perpendicular to the motion of the charged particles. Both the electric and magnetic fields exert a force on the charged particles. If a particle has precisely the right velocity, the two forces exactly cancel and the particle is not deflected. Equating the forces due to the electric field and the magnetic field gives the following equation: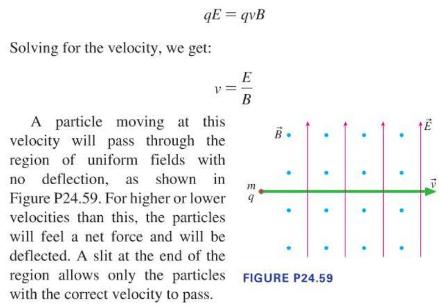
Step by Step Answer:

College Physics A Strategic Approach
ISBN: 9780321907240
3rd Edition
Authors: Randall D. Knight, Brian Jones, Stuart Field





