A simplified second-order transfer function model for bicycle dynamics is given by The input is (s), the steering angle, and the output is (s), the

A simplified second-order transfer function model for bicycle dynamics is given by

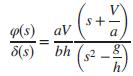
The input is δ(s), the steering angle, and the output is φ(s), the tilt angle (between the floor and the bicycle longitudinal plane). In the model parameter a is the horizontal distance from the center of the back wheel to the bicycle center of mass; b is the horizontal distance between the centers of both wheels; h is the vertical distance from the center of mass to the floor; V is the rear wheel velocity (assumed constant); and g is the gravity constant. It is also assumed that the rider remains at a fixed position with respect to the bicycle so that the steer axis is vertical and that all angle deviations are small (Åstrom, 2005).

a. Obtain a state-space representation for the bicycle model in phase-variable form.

b. Find system eigenvalues and eigenvectors.

c. Find an appropriate similarity transformation matrix to diagonalize the system and obtain the state-space system’s diagonal representation.

s+ P(s) av a 8(s) bh