Without using calculus (as in the previous exercise), show that, if n is a power of 2

Question:

Without using calculus (as in the previous exercise), show that, if n is a power of 2 greater than 1, then, for H_n, the nth harmonic number,

H_n ≤ 1 + H_n/2

Use this fact to conclude that Hn ≤ 1 + [log n], for any n ≥ 1.

Data From Previous Exercise

Use the fact that, for a decreasing integrable function, f,

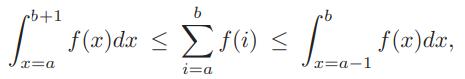
cb+1 b f(x)dx < f (x)dx, r=a r=a-1 2=a

to show that, for the nth harmonic number, H_n,

ln n ≤ H_n ≤ 1 + ln n.

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Algorithm Design And Applications

ISBN: 9781118335918

1st Edition

Authors: Michael T. Goodrich, Roberto Tamassia

See More Books

Question Posted: Aug 10, 2020 06:01 AM

See More Questions

Using the definition of a c-incremental algorithm from the previous exercise, show that, if a c-incremental algorithm A has a worst-case running time t(N) in the RAM model, as a function of the...
Consider the previous exercise, but suppose the city of Irvine, California, changed its dog-owning ordinance so that it still allows for residents to own a maximum of three dogs per household, but...
Solve the previous exercise using a queue instead of stack. That is, suppose you are given an array, A, containing n numbers in order, as in the previous exercise. Describe in pseudocode an efficient...
Suppose that in a wave of pessimism, housing prices fall by 10% across the entire economy. a. Has the stock of real assets of the economy changed? b. Are individuals less wealthy? c. Can you...
A fixed-end beam AB of length L supports a uniform load of intensity q (see figure). Beginning with the second-order differential equation of the deflection curve (the bending-moment equation),...
Consider the fraction of defective items in a batch of manufactured parts. Say that the prior distribution of is a beta distribution with parameters i = 5 and ct2 = 10 (see Section 7.6.2 for a...
Explain the nature, role, and risks involved in countertrade. LO.1
The Fisher Apparel Company balance sheet for the year ended 2006 is as follows: a. What is Fisher's investment in current assets? b. Determine Fisher's working capital investment. c. Determine...
A company using the periodic inventory system has the following account balances: Inventory at the beginning of the year, $3,838; Freight-In, $418; Purchases, $14,864; Purchases Returns and...
1. What is the cost of debt for Sunrise Bakery? 2. What is their cost of equity? 3. What is the WACC? 4. Which cost of capital should be used to evaluate the feasibility of the oven purchase? 5....
If one has a set, S, of n items, where n is even, then the median item in S is the average of the ith and (i + 1)st smallest elements in S, where i = n/2. Describe an efficient algorithm for...
What is H 5 , the 5th harmonic number?
Sound Portal Corporation sells stereos under a 2-year warranty contract that requires Sound Portal to replace defective parts and provide free labor on all repairs. During 2010, 1,290 units were sold...
Malaysian Agrifood Corporation Berhad reported sales of RM 7 0 , 0 0 0 in May and RM 8 0 , 0 0 0 in June. The forecast sales for July, August and September are RM 9 0 , 0 0 0 , RM 1 0 0 , 0 0 0 , and...
1. Refer to the \"Plotting Data\" lesson (end of "Patterns to Notice") and plot the "Skydiver Velocity vs. Time\" data (taken from the video) on the following graph. to c) d) e) f) 9) Which is the...
The Star Company is considering a change in its credit terms to increase sales. Its current credit sales are $8.5 million per year and its present credit terms are 2/16 net of 20 basis. Discount is...
Determine the shear flow distribution for a torque of 57265.0Nm applied to the three cell section shown in the figure below. Note that the section has a constant shear modulus throughout. All answers...
2 a) Figure 1 shows the orientation of the carbon fibre which is orientate at 0.A9 radians from the vertical plane. Draw and label the element's normal and shear stresses acting on the carbon fibre...
Evaluate the integral. 12 dx 4 .2
Whats the difference between an ordinary annuity and an annuity due? What type of annuity is shown below? How would you change the time line to show the other type of annuity?
Give a complete justification of Proposition 12.1.
Show that the nodes of any AVL tree T can be colored red and black so that T becomes a red-black tree.
The boolean indicator used to mark nodes in a red-black tree as being red or black is not strictly needed when we have distinct keys. Describe a scheme for implementing a red-black tree without...
A firm purchased a new piece of equipment with an estimated useful life of eight years. The cost of the equipment was $65,000. The salvage value was estimated to be $10,000 at the end of year 8....
5. Which of the following is the cheapest for a borrower? a. 6.7% annual money market basis b. 6.7% semi-annual money market basis c. 6.7% annual bond basis d. 6.7% semi-annual bond basis.
Waterloo Industries pays 30 percent corporate income taxes, and its after-tax MARR is 24 percent. A project has a before-tax IRR of 26 percent. Should the project be approved? What would your...

Without using calculus (as in the previous exercise), show that, if n is a power of 2

Question:

cb+1 b f(x)dx < f (x)dx, r=a r=a-1 2=a

Step by Step Answer:

If n is a power of 2 greater than 1 then there are n2 terms in the differe...View the full answer

Algorithm Design And Applications

Students also viewed these Computer science questions