Designs involving several Latin squares. [See Cochran and Cox (1957), John (1971).] The p × p Latin square contains only p observations for each treatment. To obtain more replications the experimenter may use several squares, say n. It is immaterial whether the squares used are the same or different. The appropriate model is

where y_ijkh is the observation on treatment j in row i and column k of the hth square. Note that α_i(h) and β_k(h) are the row and column effects in the hth square, ρ_h is the effect of the hth square, and (τp)_jh, is the interaction between treatments and squares.

(a) Set up the normal equations for this model, and solve for estimates of the model parameters. Assume that appropriate side conditions on the parameters are Σ_hρ̂_h = 0,

 

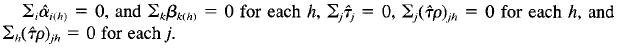
(b) Write down the analysis of variance table for this design.

Transcribed Image Text:

Σά-0 and Σβa =0 for each h Ση-0, Σ (τp). E,(tp)jh = 0 for each j. O for each h, and %3D