Consider the system of Problem 11.7-1.

(a) Suppose that all specifications are the same, except E[w( j)w(k)] = 0; that is, there are no random plant disturbances. Design a Kalman filter and estimate the steady-state Kalman gain from the trends of the calculations. Use M(0) = 2.

(b) Write the difference for the steady-state Kalman filter.

(c) In part (a), P(k) S 0 in the steady-state. Hence the errors in the estimation of the state go to zero in the steady-state (perfect estimation). Since the measurements are noisy, how can the estimation error be zero?

(d) Repeat parts (a), (b), and (c) for the case that R_w = 2 and R_v = 0. In repeating part (c), the measurements are perfect, but the plant has a random disturbance.

Problem 11.7-1

Suppose that a plant is described by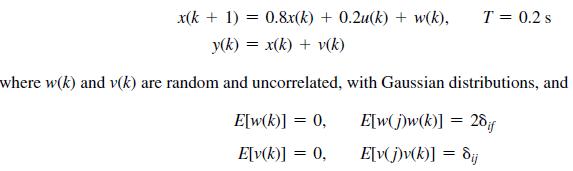

(a) Design a Kalman filter for this system. Continue the gain calculations until the gain is approximately constant. Use M(0) = 2.

(b) In part (a), we specified M(0) = 2. What are we stating about our estimate of the state x(0) ?

(c) Write the difference equations for the steady-state Kalman filter, as in part (a).

(d) Suppose that an LQ design is performed for this plant, with the resulting gain K = 0.2197. Find the control-estimator transfer function (see Fig. 9-8) for this IH-LQG design.

(e) Find the closed-loop system characteristic equation for part (d).

(f) Find the closed-loop system time constants.

(g) Suppose that the state x(k) is estimated to be 90.1 by the Kalman filter at a certain time kT. Give the three-sigma range about the value 90.1 that will almost certainly contain the true value of x(k) at that time kT.

(h) Find the system phase and gain margins.

Transcribed Image Text:

x(k+ 1) = 0.8x(k) + 0.2u(k)+ w(k), y(k)= x(k)+v(k) where w(k) and v(k) are random and uncorrelated, with Gaussian distributions, and E[w(k)] = 0, E[v(k)] = 0, T = 0.2 s E[w(j)w(k)] = 28 if E[v(j)v(k)] = 8jj