Example 4.3 calculates the step response of the system in Fig. 4-2. Example 4.4 calculates the step
Question:
Example 4.3 calculates the step response of the system in Fig. 4-2. Example 4.4 calculates the step response of the same system preceded by a digital filter with the transfer function D(z) = (2 − z−1). This system is shown in Fig. P4.4-1.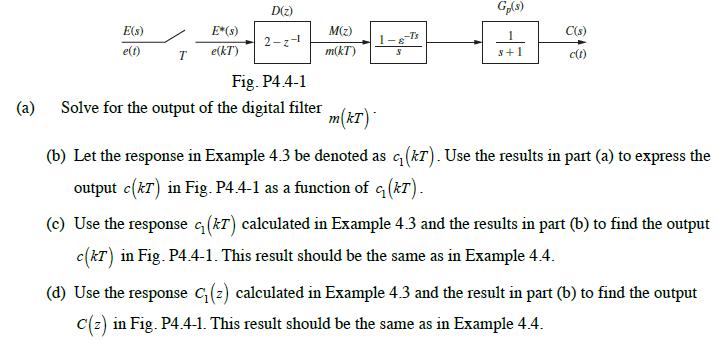
Example 4.3
Given the system shown in Fig. 4-2, with input e(t) a unit step function, let us determine the output function C(z). Now,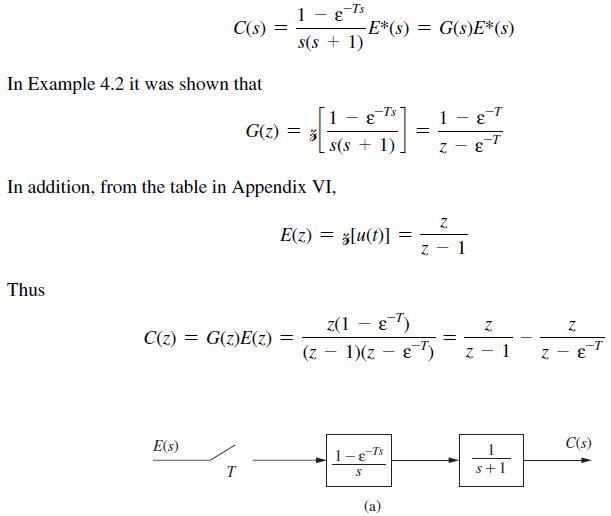
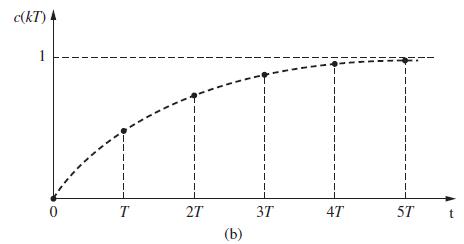
Example 4.4
Let us determine the step response of the system shown in Fig. 4-5. Suppose that the filter is described by the difference equation![]()
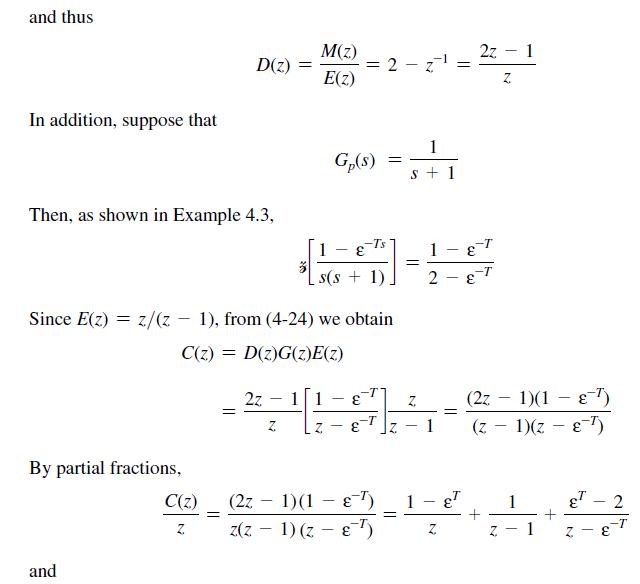
![Since C(z)= (1 - ) + [k2] = N N 1 + (T - 2)z 2- E-T n = i ki, 10, ni](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/4/7/4/85365a77b2522e231705474851379.jpg)
Figure 4-5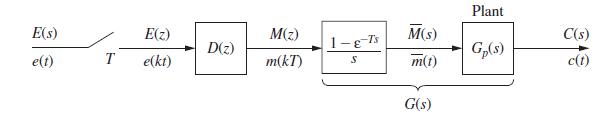
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Digital Control System Analysis And Design
ISBN: 9780132938310
4th Edition
Authors: Charles Phillips, H. Nagle, Aranya Chakrabortty
Question Posted:





