In Problem 9.2-4, a pole-placement design for a satellite control system results in the gain matrix K

Question:

In Problem 9.2-4, a pole-placement design for a satellite control system results in the gain matrix K = [0.194 0.88]. It is desired to have an input signal r(t) applied to the system, so as to realize the system of Fig. 9-12. Write the resulting state equations in the form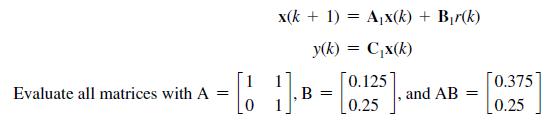 Evaluate all matrices with A = x(k+ 1) = Ax(k) + Br(k) y(k) = Cx(k) [1 B = 0.125 0.25 , and AB = [0.375 0.25

Problem 9.2-4

A satellite control system is modeled as shown in Fig. P9.2-4. This system is described in Problem 1.4-1.
For this problem, let D(z) = 1. In addition, K = 1, T = 1 s, J = 4, and H_k = 1. From the z-transform tables,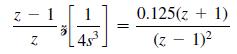 Z 1 Z 45 0.125(z + 1) (z 1)

A state model for this system is given by R(s) r(t) where x(k) is angular position and x2(k) is angular velocity. + x(k + 1) = = [61]8) + [0.25 (4) (a)