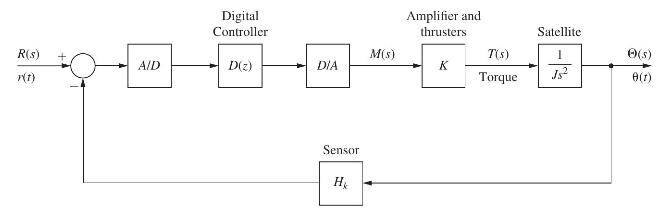
The block diagram of an attitude control system of a satellite is shown in Fig. P6.4-2. Let \(T=1 \mathrm{~s}, K=200\), \(J=0.2, H_{k}=0.04\), and \(D(z)=1\).

(a) Find the damping ratio \(\zeta\), the natural frequency \(\omega_{n}\), and the time constant \(\tau\) of the open-loop system. If the system characteristic equation has two real zeros, find the two time constants. These values can be solved by inspection. Why?

(b) Repeat part (a) for the closed-loop system.

(c) Repeat parts (a) and (b) for the system with the sampler, digital controller, and data hold removed, that is, for the analog system.

(d) The closed-loop sampled-data system is seen to be unstable and the closed-loop analog system is seen to be marginally stable. If the satellite is operated with each of these control systems, describe the resulting movement for both the sampled-data system and the analog system.

Fig. P6.4-2

Transcribed Image Text:

Digital Controller Amplifier and thrusters Satellite R(s) M(s) T(s) 1 (s) A/D D(z) DIA K r(t) Torque Js 8(r) Sensor Hk