Consider a rotor on a massless and flexible steel shaft, which is simply supported at the ends (Figure P2.6).

Figure P2.6 

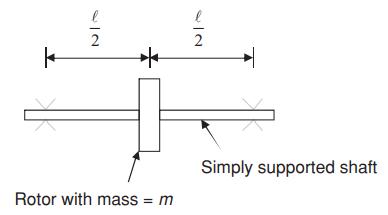
The mass of the rotor is \(10 \mathrm{~kg}\) and the eccentricity is \(0.5 \mathrm{~cm}\). The length and the diameter of the shaft are \(50 \mathrm{~cm}\) and \(5 \mathrm{~cm}\), respectively.

a. Compute the critical speed of the rotor.

b. If the rotor operates at critical speed, how much time will it take for the maximum bending stress in the shaft to be about \(70 \%\) of its yield stress? Assume that the rotor starts from rest.

c. Assuming that the rotor starts from rest, suggest an angular acceleration of the rotor to reach a speed that equals two times the critical speed. It is desired that the maximum bending stress in the shaft is below \(50 \%\) of its yield stress. Verify your result using numerical integration of differential equation.

d. For part c, plot the force transmitted to each support as a function of time.

Transcribed Image Text:

k 12 AP 2 Rotor with mass = m Simply supported shaft