Consider sampling from a multivariate normal distribution with mean vector = ( 1 , 2 , . . . , M )

Consider sampling from a multivariate normal distribution with mean vector μ = (μ₁, μ₂, . . . , μ_M) and covariance matrix σ²I. The log-likelihood function is

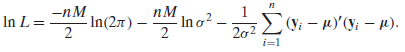
Show that the maximum likelihood estimators of the parameters are μ̂ = y̅m, and

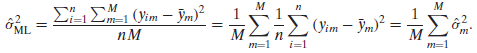
Derive the second derivatives matrix and show that the asymptotic covariance matrix for the maximum likelihood estimators is

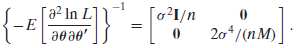
Suppose that we wished to test the hypothesis that the means of the Mdistributions were all equal to a particular value μ₀. Show that the Wald statistic would be

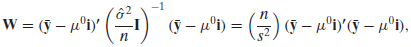
where y̅ is the vector of sample means.

-nM nM In L= 2 - In(27) "" Inc (V: p)'(yi ). 202 i=1