Consider the model in Exercise 16.7 with \(X_{t}=\widetilde{u}_{t+1}\).

a. Is the OLS estimator of \(\beta_{1}\) consistent? Explain.

b. Explain why the GLS estimator of \(\beta_{1}\) is not consistent.

c. Show that the infeasible GLS estimator \(\hat{\beta}_{1}^{G L S} \xrightarrow{p} \beta_{1}-\frac{\phi_{1}}{1+\phi_{1}^{2}}\).

Apply the omitted variable formula in Equation (6.1) to the quasi-differenced regression in Equation (16.23).

Equation (6.1)

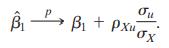
Equation (16.23)

Exercise 16.7

Consider the regression model \(Y_{t}=\beta_{0}+\beta_{1} X_{t}+u_{t}\), where \(u_{t}\) follows the stationary \(\operatorname{AR}(1)\) model \(u_{t}=\phi_{1} u_{t-1}+\widetilde{u}_{t}\) with \(\widetilde{u}_{t}\) i.i.d. with mean 0 and variance \(\sigma_{\tilde{u}}^{2}\) and \(\left|\phi_{1}\right|

B BPxu