Consider a teacher who has to divide her time between at least two activities: teaching and mentoring her students. The quality of her students depends on the number of hours that she spends with them, both teaching and mentoring. The quality function is: q = x · y, where x is the time that the teacher spends teaching, and y is the time that she spends mentoring her students each day. Each day, the teacher can work for only ten hours. Suppose that the principal of the school has a utility function that depends on the quality of her students: u = q. The principal can verify teaching activities via teaching evaluations: bad, enough, good, or excellent. (She can observe the time that the teacher is working, but can not fully verify how the teacher allocates her time between teaching preparation and mentoring.) Suppose that in order to attain decent teaching evaluations, the teacher has to spend time

(and her salary ultimately depends on this time) as illustrated in the following table:

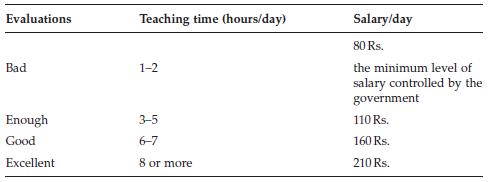
Assuming that one hour of teaching per day costs the teacher ten rupees, while one hour of mentoring costs seven rupees, and that the teacher is risk-neutral. (She just wants to maximize her net revenue.)
Compute optimal time allocation for both the teacher and the principal.
In what way does your answer relate to the problem confronted by managers of MFIs?

Transcribed Image Text:

Evaluations Bad Teaching time (hours/day) 1-2 Salary/day 80 Rs. the minimum level of salary controlled by the government 110 Rs. 160 Rs. Enough 3-5 Good 6-7 Excellent 8 or more 210 Rs.