Gray€™s Gravel and Gravel Depot are duopoly producers of gravel in a small city. Industry output Q is the sum of Gray€™s output and Gravel Depot€™s output. The market demand function for truckloads of gravel isQ = 20p^ˆ’2.0 and therefore has a constant price elasticity ofˆ’2.The inverse market demand function isp = (20/Q)^0.5.As in the Cournot model of Chapter 11, the firms decide simultaneously on their output levels, but each firm has only three possible output choices: 1, 2, or 3 truckloads. Each firm has a constant average cost and marginal cost of 7. 

a. In an Excel spreadsheet, create labels at the top of columns A through J indicating, in order, Gray€™s output, Gravel Depot€™s output, industry output, industry price, the revenue for each of the firms, the cost for each of the firms, and each firm€™s profit. In Column A, enter the numbers 1, 2, and 3 in cells A2, A3, and A4, then again in cells A5 €“ A7 and again in cells A8 €“ A10. In column B enter the number 1 in cells B2 €“ B4, the number 2 in cells B5 €“ B7, and the number 3 in cells B8 €“ B10. Fill in the corresponding formulas and determine the values in the other columns for each possible output combination (rounded to one digit after the decimal point).

b. In the same spreadsheet, create the game€™s profit matrix as follows. Enter the numbers 1, 2, and 3 in cells B16 €“ B18. These numbers are the possible output levels for Gray€™s Gravel. Enter the numbers 1, 2, and 3 in cells C15 €“ E15, showing the possible output levels for Gravel Depot. Then enter the firms€™ profit levels in cells C16 €“ E18. Follow the convention illustrated in Question 7.1: For each cell (that is, for each output combination) enter the profit of Gray€™s, followed by a comma, followed by the profit of Gravel Depot. What is the Nash equilibrium in this game?

c. If firms could produce 4 units of output instead of being limited to a maximum of 3, would that change the Nash equilibrium?

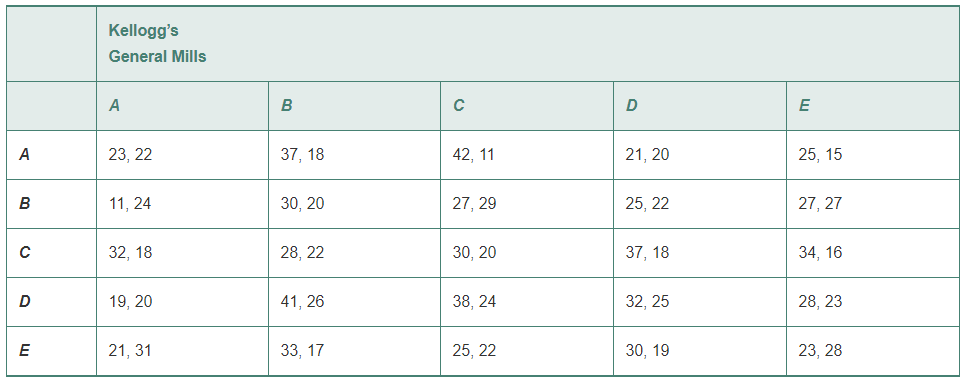
Question 7.1

General Mills and Kellogg€™s, major rivals in the breakfast cereal market, decide simultaneously on their advertising strategies. Each has five options, A through E. The following table shows their net profits (in millions of dollars) for various advertising strategy combinations. In each cell, the first entry is the profit for Kellogg€™s and the second is the profit for General Mills. 

Transcribed Image Text:

Kellogg's General Mills A 23, 22 37, 18 21, 20 25, 15 42, 11 27, 29 30, 20 25, 22 27, 27 11, 24 28, 22 30, 20 37, 18 34, 16 32, 18 38, 24 32, 25 19, 20 41, 26 28, 23 33, 17 25, 22 30, 19 21, 31 23, 28