An infinite elastic medium IzI ≥ a is bonded over its internal boundary IzI =a to a rigid inclusion of radius a. The inclusion is acted upon by a force X + iY and a moment Mabou its center. Show that the problem is solved by the potentials:

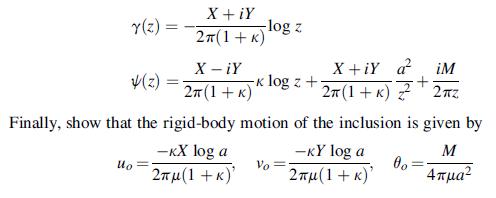
Transcribed Image Text:

Y(z) = = Uo X +iY 2(1+K) X-iY 2(1+K) -log z -K log z + X +iY a 2 (1+K) 2 Finally, show that the rigid-body motion of the inclusion is given by -KX log a -KY log a M 00: 2(1 +)' 2(1 + x)' Vo iM 2z