Rework Example 6.2 using the trigonometric Ritz approximation w j = sin jx/l. Develop a two-term approximate solution and compare it with the displacement solution

Rework Example 6.2 using the trigonometric Ritz approximation w_j = sin jπx/l. Develop a two-term approximate solution and compare it with the displacement solution developed in the text. Also compare each of these approximations with the exact solution (6.7.9) at midspan x = l/2.

Data from example 6.2

Consider a simply supported Euler-Bernoulli beam of length l carrying a uniform loading q_o. This one-dimensional problem has displacement boundary conditions:

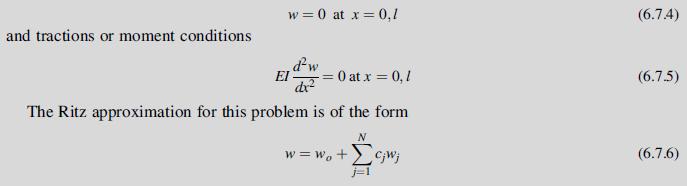
With no nonhomogeneous boundary conditions, w_o = 0. For this example, we choose a polynomial form for the trial solution. An appropriate choice that satisfies the homogeneous conditions (6.7.4) is w_j = x ^j (l – x). Note this form does not satisfy the traction conditions (6.7.5). Using the previously developed relation for the potential energy (6.6.12), we get:

Equation 6.7 .4

Equation 6.7 .5

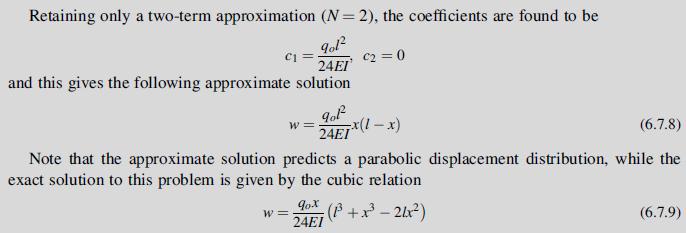
Actually, for this special case, the exact solution can be obtained from a Ritz scheme by including polynomials of degree three.

and tractions or moment conditions w = 0 at x = 0,1 dw dx The Ritz approximation for this problem is of the form w = wo +Cjwj = = 0 at x = 0, / (6.7.4) (6.7.5) (6.7.6)