Show that the distortional strain energy given by (6.1.17) is related to the octahedral shear stress (3.5.4)
Question:
Show that the distortional strain energy given by (6.1.17) is related to the octahedral shear stress (3.5.4)2 by the relation:
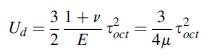
Equation 6.1 .17
![Ua 121 [(x a) + (ay 0) + (0 0x) + 6(y + 3 +2)] -](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1704/9/5/6/537659f9279ad1e31704956536642.jpg)
Equation 3.5 .4
![N = 0 oct -3 (0 + 0 +03) = 30kk = 3/1 S = Toct = 1/[(0-0) + (0-03) + (03-0) ] /2 = (217-612) /2](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1704/9/5/6/577659f92a1ec6101704956576888.jpg)
Results from Exercise 3.5 may be helpful.
Data from exercise 3.5
A two-dimensional state of plane stress in the x, y-plane is defined byσz = τ yz = τ zx = 0. Using general principal value theory, show that for this case the in-plane principal stresses and maximum shear stress are given by:
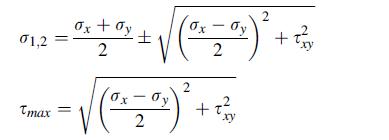
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Elasticity Theory Applications And Numerics
ISBN: 9780128159873
4th Edition
Authors: Martin H. Sadd Ph.D.
Question Posted:





