Under the conditions of polar axis symmetric, verify that the Navier equations (5.4.4) reduce to relation (8.3.10).
Question:
Under the conditions of polar axis symmetric, verify that the Navier equations (5.4.4) reduce to relation (8.3.10). Refer to Example 1.5 to evaluate vector terms in (5.4.4) properly. Next show that the general solution to this CauchyeEuler differential equation is given by (8.3.11). Finally, use this solution to determine the stresses and show that they will not contain the logarithmic terms given in the general solution (8.3.8).
Data from example 1.5
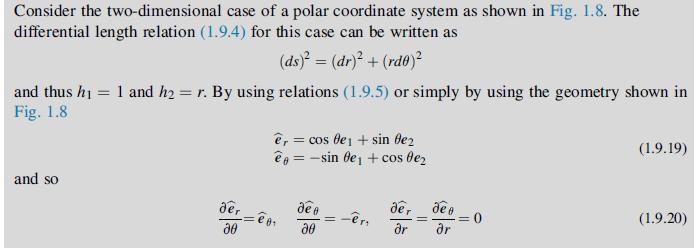
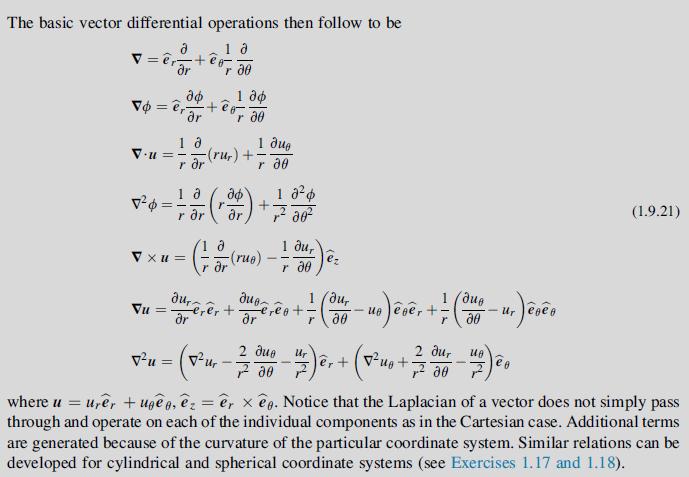
Equation 5.4.4
![]()
Equation 8.3.10
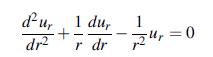
Equation 8.3.11
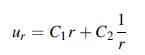
Equation 8.3.8
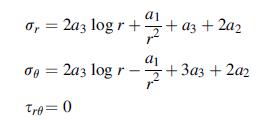
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Elasticity Theory Applications And Numerics
ISBN: 9780128159873
4th Edition
Authors: Martin H. Sadd Ph.D.
Question Posted:





