Using the solution from Example 10.7, apply the principle of superposition and solve the problem of a
Question:
Using the solution from Example 10.7, apply the principle of superposition and solve the problem of a stress-free elliptical hole under uniform biaxial tension as shown. In particular show that the circumferential stress is given by:
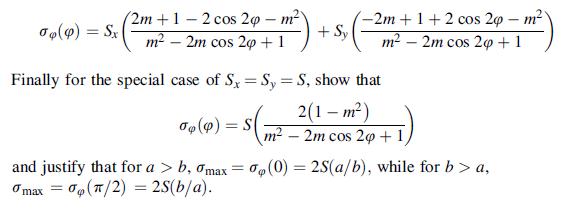
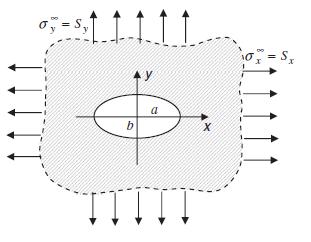
Data from example 10.7
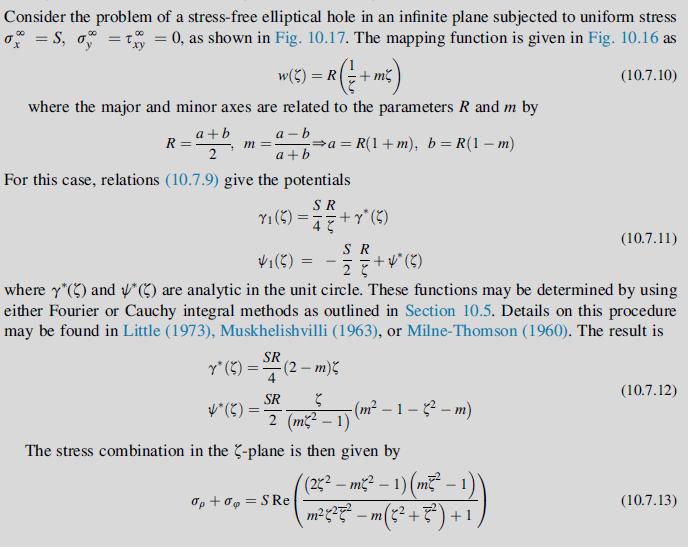
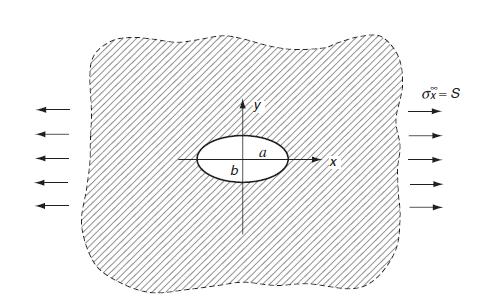
Transcribed Image Text:
Jp (9) = Sx (2m+1-2 cos 2p - m m 2m cos 20+1 Finally for the special To (9)= S( case of Sx = Sy = S, show that 2(1-) 2m cos 2p+1, = and justify that for a> b, max: max = 0,(1/2) = 25(b/a). m + Sy - -2m+1+2 cos 2q - m m - 2m cos 2p+1 op (0)= 2S(a/b), while for b> a,
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 40% (5 reviews)
Applying St b 1 Horizontal Loading b Sx o S AV b a 2 Vertical Loading 0 0 Sx q S 2 2m 12 cos 20 m ...View the full answer

Answered By

Parvesh Kumar
I am an experienced Mathematics and Statistics tutor with 10 years of experience teaching students and working professionals. I love teaching students who are passionate to learn subjects or wants to understand any mathematics and statistics concept at graduation or master’s level. I have worked with thousands of students in my teaching career. I have helped students deal with difficult topics and subjects like Calculus, Algebra, Discrete Mathematics, Complex analysis, Graph theory, Hypothesis testing, Probability, Statistical Inference and more. After learning from me, students have found Mathematics and Statistics not dull but a fun subject. I can handle almost all curriculum of mathematics. I did B.Sc (mathematics), M.Sc (mathematics), M.Tech (IT) and am also Gate (CS) qualified. I have worked in various college and school and also provided online tutoring to American and Canadian students. I look forward to discussing with you and make learning a meaningful and purposeful
5.00+
4+ Reviews
10+ Question Solved
Related Book For 

Elasticity Theory Applications And Numerics
ISBN: 9780128159873
4th Edition
Authors: Martin H. Sadd Ph.D.
Question Posted:
Students also viewed these Engineering questions
-
Using the results of Exercise 13.20, continue the superposition process by combining three force doublets in each of the coordinate directions. This results in a center of dilatation at the origin as...
-
A force doublet is commonly defined as two equal but opposite forces acting in an infinite medium as shown in the following figure. Develop the stress field for this problem by superimposing the...
-
A parallel-plate capacitor consists of two flat metal plates of area A separated by a small distance d. The plates are given equal and opposite net charges q. (a) Sketch the field lines and use your...
-
In the game of roulette, a gambler who wins the bet receives $36 for every dollar she or he bet. A gambler who does not win receives nothing. If the gambler bets $1, what is the expected value of the...
-
A block is on a horizontal surface (a shake table) that is moving back and forth horizontally with simple harmonic motion of frequency 2.0 Hz. The coefficient of static friction between block and...
-
What is an authoritative page? What is a hub? What is the difference between the two?
-
6. The class should also consider supervisors efforts to take corrective action. Did supervisors intervene too much, or not enough? How did supervisors attempts help or hurt employees efforts? What...
-
Prepare the statement of retained earnings for Damon Design Studio for the year ending December 31, 2018. The assets, liabilities, and equities of Damon Design Studio have the following balances at...
-
Exercise 6-04 Sage Hill Inc, sells a snowboard, EZ slide, that is popular with snowboard enthusiasts. Below is Information relating to Sage Hill Inc.'s purchases of EZslide snowboards during...
-
Consider the problem of an infinite plate containing a stress-free elliptical hole with x = y = 0; xy = S. For this problem, the derivative of the complex potential has been developed by...
-
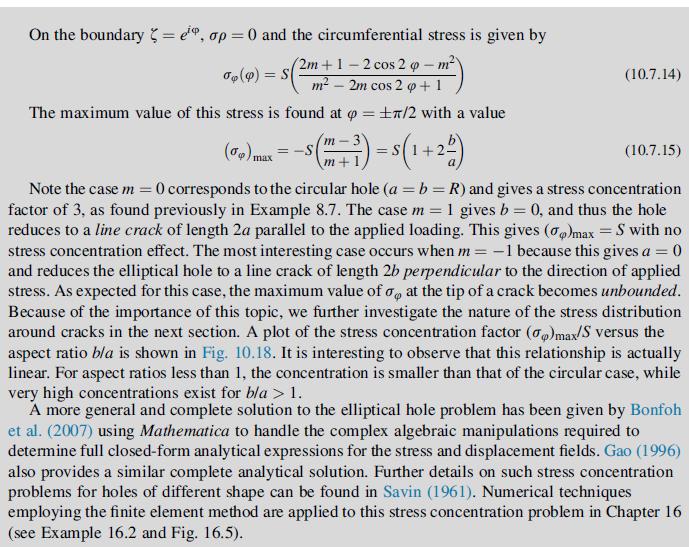
Consider relation (10.7.14) for the circumferential stress on the boundary of the elliptical hole shown in Fig. 10.17. Explicitly verify that the maximum stress occurs at = /2. Next plot the...
-
Mandy Robertson has just bought a new house and wants to consolidate her finances. She has three loans outstanding. They all mature in 5 years, and she can repay them without penalty any time before...
-
Evaluate the following definite integrals as limit of sums: 1. (x (x-x)dx [(2x 2. (2x+5x)dx 3. (2x + +3x+1)dx
-
Evaluate as limit of sums Jr- 3+1dx 1
-
1. 3. Example: Evaluate the following definite integrals as limit of sums: e'dx fedx 2. Je dx 4.
-
Evaluate the following definite integrals as limit of sums: 1. 2 2. 5*x
-
Evaluate the following definite integrals as limit of sums: 1. sin sinxdx 2. 0 /4 cos.xdx 3. sin x dx 2/6
-
m(t) = 1 - t. Suppose a population of bacteria grows according to P(t) = 10et. Find the first and second derivative to graph the total mass when the mass per individual m(t) has the following forms....
-
The following selected information was taken from Sun Valley Citys general fund statement of revenues, expenditures, and changes in fund balance for the year ended December 31, 2019: Revenues:...
-
An engineer determines that a 40-cm-long rod of 1020 grade steel will be subjected to a tension of 20 kN. The following two design requirements must be met: The stress must remain below 145 MPa, and...
-
Find a real physical example of a mechanical structure or machine that has shear stress present. (a) Make a clear, labeled drawing of the situation. (b) Estimate the dimensions of the structure or...
-
Adhesive tape is capable of supporting relatively large shear stress, but it is not able to support significant tensile stress. In this problem, you will measure the shear strength of a piece of...
-
*Prepare the plant assets section of Amphonie's balance sheet at December 31, 2021 using the information below. At December 31, 2020, Amphonie Company reported the following as plant assets. Land $...
-
Question 1 of 1 - / 100 View Policies Current Attempt in Progress Pargo Company is preparing its budgeted income statement for 2020. Relevant data pertaining to its sales, production, and direct...
-
Schopp Corporation makes a mechanical stuffed alligator that sings the Martian national anthem. The following information is available for Schopp Corporation's anticipated annual volume of 500,000...

Study smarter with the SolutionInn App


