Verify the validity of the transformation relations given in Table 7.1 by: (a) Transforming the plane strain
Question:
Verify the validity of the transformation relations given in Table 7.1 by:
(a) Transforming the plane strain Hooke’s law (7.1.3) into the corresponding plane stress results given in Exercise 7.6 .
(b) Transforming the plane stress Hooke’s law (7.2.2) into the corresponding plane strain results given in Exercise 7.1 .
Table 7.1
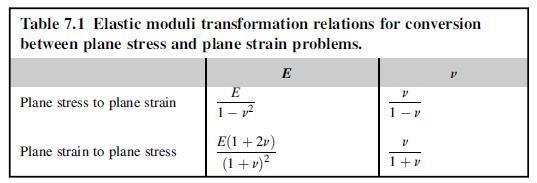
Equation 7.1 .3
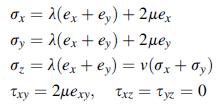
Data from exercise 7.6 Invert the plane stress form of Hooke’s law (7.2.2) and express the stresses in terms of the strain components:
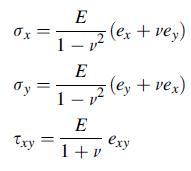
Equation 7.2 .2
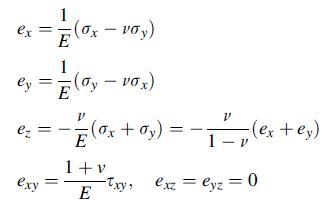
Data from exercise 7.1 Invert the plane strain form of Hooke’s law (7.1.3) and express the strains in terms of the stresses as:
![ex ey exy - 1 + ((1 - vox - voy] E 1+v E [(1 - v)oy - vox] 1 + v E -Txy](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1704/9/6/2/183659fa887c126d1704962182359.jpg)
Equation 7.13
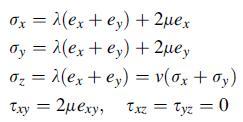
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Elasticity Theory Applications And Numerics
ISBN: 9780128159873
4th Edition
Authors: Martin H. Sadd Ph.D.
Question Posted:





