1.*16. Let {Xn,S?;;} be a martingale: Xl = Xl, Xn = Xn -Xn-l for n :::. 2;

let Vn E 3?;;-1 for n :::. 1 where.% = .9l; now put j=l

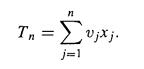Show that {Tn, g;;} is a martingale provided that Tn is integrable for every n.
The martingale may be replaced by a smartingale if Vn > 0 for every n. As a particular case take Vn 1 {nsa} where a is an optional r.v. relative to {¥n}.
What then is Tn? Hence deduce the Corollary to Theorem 9.3.4.

Transcribed Image Text:

In n =vjxj. j=1