Euro Exchange Rates. The exchange rate that we consider is the amount of euros that one can purchase for one U.S. dollar. We have \(T=699\) daily observations from the period April 1, 2005, through January 8, 2008. The data were obtained from the Federal Reserve (H10 report). The data are based on noon buying rates in New York from a sample of market participants and they represent rates set for cable transfers payable in the listed currencies. These are also the exchange rates required by the Securities and Exchange Commission for the integrated disclosure system for foreign private issuers.

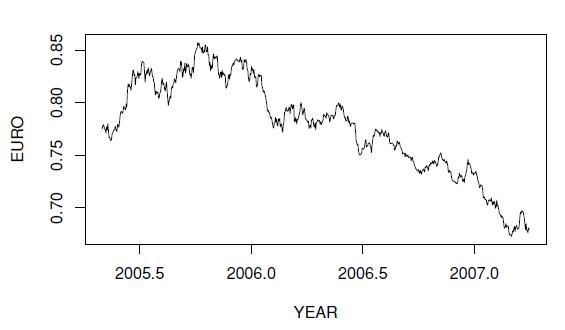
a. Figure 7.10 is a time series plot of the Euro exchange rate.
a(i). Define the concept of a stationary time series.
a(ii). Is the EURO series stationary? Use your definition in part a(i) to justify your response.

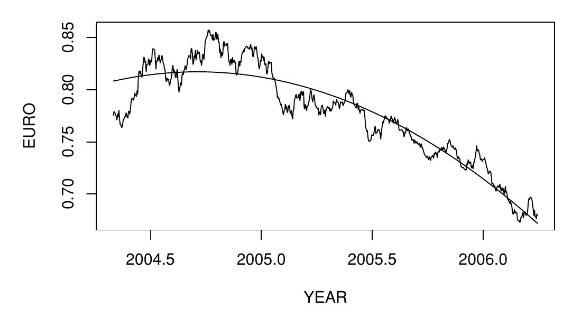
b. After an inspection of Figure 7.10 in part (a), you decide to fit a quadratic trend model of the data. Figure 7.11 superimposes the fitted value on a plot of the series.
b(i). Cite several basic regression statistics that summarize the quality of the fit.
b(ii). Briefly describe any residual patterns that you observe in Figure 7.11.

b(iii). Here, TIME varies from 1, 2, . ., 699. Using this model, calculate the three-step forecast corresponding to TIME \(=702\).

c. To investigate a different approach, DIFFEURO, calculate the difference of EURO. You decide to model DIFFEURO as a white noise process.
c(i). What is the name for the corresponding model of EURO?
c(ii). The most recent value of EURO is \(E U R O_{699}=0.6795\). Using the model identified in part c(i), provide a three-step forecast corresponding to TIME \(=702\).
c(iii). Using the model identified in part c(i) and the point forecast in part c(ii), provide the corresponding \(95 \%\) prediction interval for \(\mathrm{EURO}_{702}\).

Transcribed Image Text:

EURO 0.70 0.75 0.80 0.85 2005.5 2006.0 2006.5 2007.0 YEAR