Prove that the generalized logit model (5.6) can be defined with utility functions (y_{i j}^{*}=alpha_{j}+boldsymbol{beta}_{j}^{top} mathbf{x}+varepsilon_{i j}),
Question:
Prove that the generalized logit model (5.6) can be defined with utility functions \(y_{i j}^{*}=\alpha_{j}+\boldsymbol{\beta}_{j}^{\top} \mathbf{x}+\varepsilon_{i j}\), where \(\varepsilon_{i j}\) follows the standard Gumbel distribution for each level \(j=1, \ldots, J\) and for subjects \(i=1, \ldots, n\), and that \(\left\{\varepsilon_{i, 1}, \ldots, \varepsilon_{i, \mathrm{~J}}\right\}\) are independent.
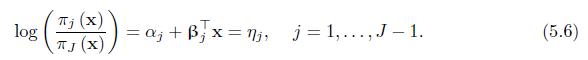
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Applied Categorical And Count Data Analysis
ISBN: 9780367568276
2nd Edition
Authors: Wan Tang, Hua He, Xin M. Tu
Question Posted:





