Construct a 99% confidence interval for the difference between the mean annual salaries of entry level architects
Question:
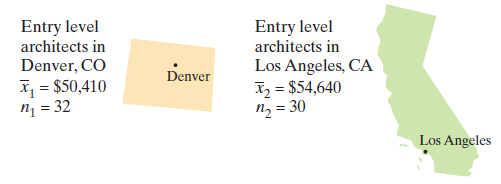
Construct a 99% confidence interval for the difference between the mean annual salaries of entry level architects in Denver, Colorado, and Los Angeles, California, using the data from Exercise 28.
You can construct a confidence interval for the difference between two population means μ1 - μ2, as shown below, when both population standard deviations are known, and either both populations are normally distributed or both n1 ≥ 30 and n2 ≥ 30. Also, the samples must be randomly selected and independent.
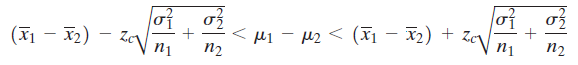
Construct the indicated confidence interval for μ1 - μ2.
Data from exercise 28:
Is the difference between the mean annual salaries of entry level architects in Denver, Colorado, and Los Angeles, California, equal to $10,000? To decide, you select a random sample of entry level architects from each city. The results of each survey are shown in the figure. Assume the population standard deviations are σ1 = $6520 and σ2 = $7130. At α = 0.01, what should you conclude?
Step by Step Answer:

Elementary Statistics Picturing The World
ISBN: 9780134683416
7th Edition
Authors: Ron Larson, Betsy Farber





