Construct a 95% confidence interval for the difference between the mean annual salaries of entry level software engineers in Raleigh, North Carolina, and Wichita, Kansas, using the data from Exercise 27.

You can construct a confidence interval for the difference between two population means μ₁ - μ₂, as shown below, when both population standard deviations are known, and either both populations are normally distributed or both n₁ ≥ 30 and n₂ ≥ 30. Also, the samples must be randomly selected and independent.

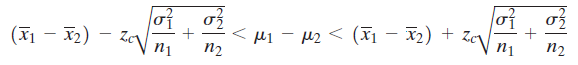
Construct the indicated confidence interval for μ₁ - μ₂.

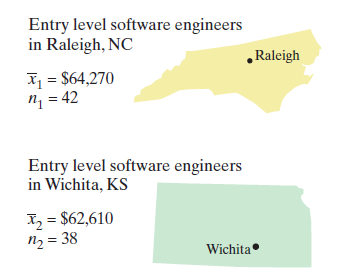
Data from Exercise 27:

Is the difference between the mean annual salaries of entry level software engineers in Raleigh, North Carolina, and Wichita, Kansas, more than $2000? To decide, you select a random sample of entry level software engineers from each city. The results of each survey are shown in the figure at the left. Assume the population standard deviations are σ₁ = $10,850 and σ₂ = $10,970. At α = 0.05, what should you conclude?

Transcribed Image Text:

σ σ σ V n1 <μ μω< ( ) + ( Ει ) Ζεy n2 n2 Entry level software engineers in Raleigh, NC Raleigh X1 = $64,270 nį = 42 Entry level software engineers in Wichita, KS I, = $62,610 n2 = 38 Wichita