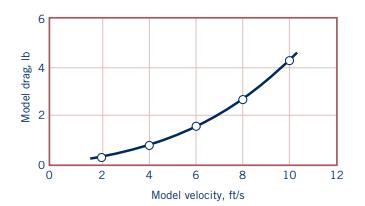
The drag on a sphere moving in a fluid is known to be a function of the sphere diameter, the velocity, and the fluid viscosity and density. Laboratory tests on a 4-in.-diameter sphere were performed in a water tunnel and some model data are plotted in Fig. P7.66.

For these tests the viscosity of the water was \(2.3 \times 10^{-5} \mathrm{lb} \cdot \mathrm{s} / \mathrm{ft}^{2}\) and the water density was \(1.94 \mathrm{slugs} / \mathrm{ft}^{3}\). Estimate the drag on an 8 - \(\mathrm{ft}\)-diameter balloon moving in air at a velocity of \(3 \mathrm{ft} / \mathrm{s}\). Assume the air to have a viscosity of \(3.7 \times 10^{-7} \mathrm{lb} \cdot \mathrm{s} / \mathrm{ft}^{2}\) and a density of \(2.38 \times 10^{-3}\) slugs \(/ \mathrm{ft}^{3}\).

Figure P7.66

Transcribed Image Text:

6 10 + Model drag, lb 2 0 0 2 4 6 8 10 12 Model velocity, ft/s