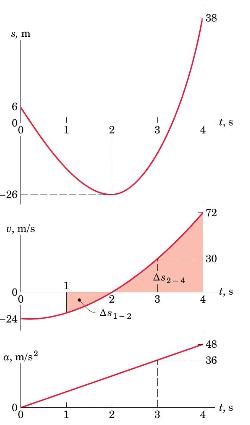
The position coordinate of a particle which is confined to move along a straight line is given by \(s=2 t^{3}-24 t+6\), where \(s\) is measured in meters from a convenient origin and \(t\) is in seconds. Determine \((a)\) the time required for the particle to reach a velocity of \(72 \mathrm{~m} / \mathrm{s}\) from its initial condition at \(t=0,(b)\) the acceleration of the particle when \(v=30 \mathrm{~m} / \mathrm{s}\), and \((c)\) the net displacement of the particle during the interval from \(t=1 \mathrm{~s}\) to \(t=4 \mathrm{~s}\).

Transcribed Image Text:

8, m 38 -26 u, m/s -24 a, m/s2 0 0 1 2 1 00 As24 72 1,5 30 1, s 2 3 4 As1-2 48 36 1 t, s 2 3 4